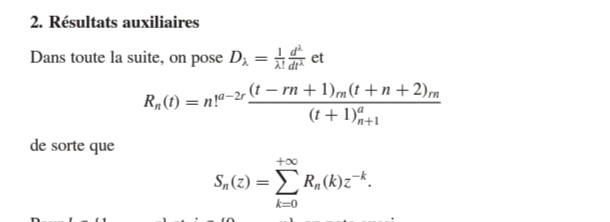I am self studying a research paper in analytic number theory (Ball and Rivoal - Irrationalité d’une infinité de valeurs de la fonction zêta aux entiers impairs) and I am unable to think about an argument in 1 of it's lemma.
Note - This question was earlier asked on MSE on 18 March but no one answered it, so I am asking it here. (https://math.stackexchange.com/questions/3584106/unable-to-think-about-argument-involving-integratibility-of-a-function-in-a-rese)
The paper is in French but I am adding images of formulas references only. Rest of text which could be useful I will write. (For more details see 7th page, logical p. 199, of research paper.)
Assume $\tilde S(z)$ to be RHS of equation 8.
Assume that it has been proved $\tilde S(z)$ is continuous on $E$ and it has also been proved that $S_n(z)$ is continuous on $E$ where $E =\{z \in \mathbb C : \lvert z\rvert \geq1\}$. Also, $a$, $b$, $n$ are integers and $1\leq b \leq a$ and $1 \leq r < a/2$.
I am not able to think why they must be equal on the given domain. On one side is an infinite series and on other side an integral with some other terms.
Can someone please help, I have no clue regarding this and no further explanation has been given in the research paper.