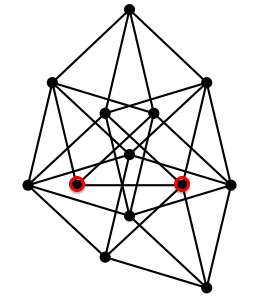
Following up on Gerry Myerson's suggestion, the following graph from Ágoston and Pálvölgyi's Improved constant factor for the unit distance problem may be a counterexample.
This is the $n=13$ example illustrating the maximum number (30) of unit distances among 13 points in the plane. Schade's 1993 thesis establishes that this is the unique maximal "unit distance graph" for 13 points. Looking at the 3 neighbors of the lefthand marked vertex $x$ and the 5 neighbors of the righthand marked vertex $y$, you can see that there is no vertex $z$ adjacent to both $x$ and $y$.