From a previous discussion here Origin of the convolution theorem, it was shown that the property of convolution $y(t)$=$a$*$b$ becoming a multiplication after Fourier transform: $F$$(y(t))$= $F(a)F(b)$, was well known by early 1900s and clearly mentioned in 1941.
I was searching the earliest known use of deconvolution by Fourier transforms. Surprisingly, the term deconvolution is quite recent as per the unabridged version of the Oxford English Dictionary (OED). In deconvolution, two functions are divided in the Fourier domain to recover the original function, say $a$, if $y(t)$ and $b(t)$ are known. For example, if we wish to recover $a$, we can divide $F(y(t))$ by $F(b)$ and do an inverse transform to get $a$. It may not be a rigorous way, but it is a popular technique in spectroscopy from an empirical perspective.
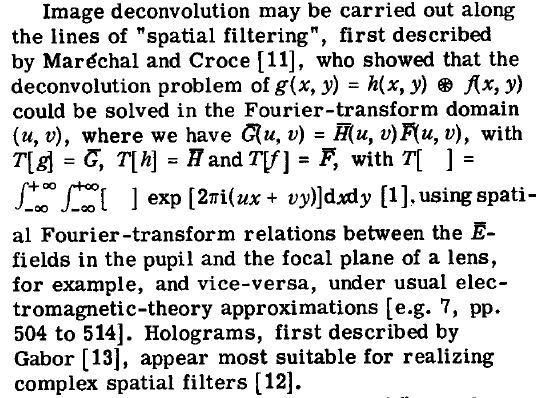
OED mentions a 1967 paper titled "Posteriori image-correcting "deconvolution" by holographic fourier-transform division" in Physics Letters. The authors show the following:
The authors cite Maréchal and Croce as the first example in Comptes Rendus, however Gallica Original Paper does not have a single equation and the word Fourier is mentionmentioned only in the first two lines! So this reference seems to be incorrect.
I am not interested in image analysis, but rather in the earliest known use of division process in the Fourier domain to recover original functions.
a) I wanted to know if mathematicians were using this approach well before 1960s to recover an original function from given convolution?
b) Spectroscopists call the division in the Fourier domain as deconvolution, what do mathematician call the process of division of two functions in the Fourier domain?
Update (30 Mar 2020)
From the detailed response by Tom Copeland, and the Table 1 shown in History of Convolution one can see another reference from 1943,
G. Doetsch, Theorie und Anwendung der Laplace-Transformation. New York: Dover, 1943
and the note 200 reads:
The reference to Picherle is given as "I. Studi sopra alcune operazioni funzionali. Mem. Accad. Bologna (4) 7 (1886)."
However the Table 1 of the convolution history mentions 1907. No reference is provided.
Thanks.