I want to apply [EGA IV 4,Proposition 17.13.2.][1] to a cartesian diagram [![enter image description here][2]][2] of algebraic spaces over a fixed scheme $S$.
I know the relative dimensions $\mathrm{dim}(\mathfrak{X}'/S),\mathrm{dim}(\mathfrak{Y}/S)$ and $\mathrm{dim}(\mathfrak{X}/S)$ and I want to use the Proposition to determine the relative dimension of $\mathfrak{Y}'$ by showing that the map on tangent spaces is surjective.
The motivation for my question is [L.Lafforgue, Proposition 1, page 29][3] where he obviously applies the Proposition from EGA to a diagram of algebraic spaces.
The problem that I am concerned about is, that the Proposition is formulated and proven only for the case of schemes, so a priori it is not immediately applicable to the diagram.
$\DeclareMathOperator\dim{dim}$I want to apply EGA IV 4, Proposition 17.13.2 to a cartesian diagram
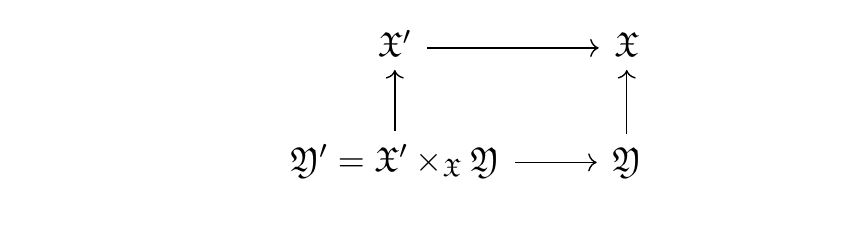 of algebraic spaces over a fixed scheme $S$.
of algebraic spaces over a fixed scheme $S$.
I know the relative dimensions $\dim(\mathfrak{X}'/S)$, $\dim(\mathfrak{Y}/S)$ and $\dim(\mathfrak{X}/S)$ and I want to use the Proposition to determine the relative dimension of $\mathfrak{Y}'$ by showing that the map on tangent spaces is surjective.
**$\bullet$ State and prove the Lemma for algebraic spaces.**(If it still holds?)The motivation for my question is L. Lafforgue, Proposition 1, page 29 where he obviously applies the Proposition from EGA to a diagram of algebraic spaces.
How is the tangent space of an algebraic space even defined? Is this the tangent space of a functor as defined in deformation theory?The problem that I am concerned about is, that the Proposition is formulated and proven only for the case of schemes, so a priori it is not immediately applicable to the diagram.
**$\bullet$ Use the fact, that every algebraic space has an atlas and apply the lemma to the resulting diagram!?**I expect at least one of the following ideas to work:
I could not figure any of these ideas out yet and would appreciate, if someone could explain me what the author had in his mind.State and prove the Lemma for algebraic spaces. (If it still holds?) How is the tangent space of an algebraic space even defined? Is this the tangent space of a functor as defined in deformation theory?
Use the fact that every algebraic space has an atlas and apply the lemma to the resulting diagram. I could not figure any of these ideas out yet and would appreciate, if someone could explain me what the author had in his mind.
