The Mehta integral is the following expression:
$$\frac{1}{(2\pi)^{n/2}}\int_{-\infty}^{\infty} \cdots \int_{-\infty}^{\infty} \prod_{i=1}^n e^{-t_i^2/2} \prod_{1 \le i < j \le n} |t_i - t_j |^{2 \gamma} dt_1 \cdots dt_n =\prod_{j=1}^n\frac{\Gamma(1+j\gamma)}{\Gamma(1+\gamma)}.$$
To simplify the notation, we introduce a measure $$d\mu_{n,\gamma}(t):=\prod_{i=1}^n e^{-t_i^2/2} \prod_{1 \le i < j \le n} |t_i - t_j |^{2 \gamma} \ dt. $$ It is easy to see that $1$ is orthogonal to $(t^2-1)$ when weighted with a Gaussian measure \begin{equation} \label{eq:ortho} \frac{1}{(2\pi)^{1/2}}\int_{-\infty}^{\infty} e^{-t^2/2} (t^2-1)dt =0. \end{equation}
Now, it seems that again for different scalings of $\gamma$ we find interesting phenomena for the value of
$$\nu:=\lim_{n \rightarrow \infty}\frac{\int (t_1^2-1) \ d\mu_{n,\gamma}(t)}{\sqrt{\int (t_1^2-1)^2 \ d\mu_{n,\gamma}(t)}}$$$$\nu:=\lim_{n \rightarrow \infty}\frac{\int (t_1^2-1) \ d\mu_{n,\gamma}(t)}{\sqrt{\int (t_1^2-1)^2 \ d\mu_{n,\gamma}(t)}\sqrt{\int 1 \ d\mu_{n,\gamma}(t)}}$$
where I admit that I use the limit without actually knowing whether it exists.
Case 1:
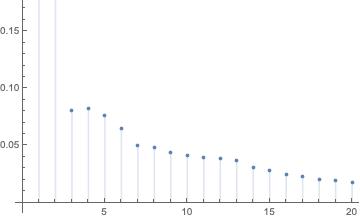
As one can guess from this thread, if we choose $\gamma=1/n^2$ it seems that the product $F(t)$ does not contribute to the value of the above limit and we have $\nu=0.$
Case 2:
If we choose $\gamma=1/n$ then Carlo Beenakker's answer that treats the case 3 rigorously suggests that we find $\nu=0$ in this case, too.
Case 3:
If we choose $\gamma=1$ then it seems like we get that $\nu$ is of order one, which is confirmed by Carlo Beenakker's answer.
My question is: Consider Case 2 with scaling $\gamma=1/n$, then find the value of $\nu$ for large $n$?