Is there a way to define perverse sheaves categorically/geometrically ? Definitions like the following from lectures by Sophie Morel:
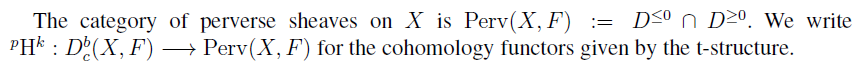
The category of perverse sheaves on $X$ is $\mathrm{Perv}(X,F):=D^{\leq 0} \cap D^{\geq 0}$. We write $^p\mathrm{H}^k \colon D_c^b(X,F) \to \mathrm{Perv}(X,F)$ for the cohomology functors given by the $\mathrm{t}$-structure.
are well and good, but to me, it feels like they come with too many "luggages", and therefore make me feel like I don't have as good of an intuition of derived categories as I should.
