Every simple group below are assumed non-abelian.
Let us call the class number $k(G)$ of a finite group $G$ the number of its conjugacy classes (also, the number of its irreducible complex representations, up to equivalence). Here is the computation of the order and the class number of the first finite simple groups:
gap> it:=SimpleGroupsIterator(10,1000);
<iterator>
gap> for G in it do Print([G,Order(G),NrConjugacyClasses(G)]); od;
[ A5, 60, 5 ][ PSL(2,7), 168, 6 ][ A6, 360, 7 ][ PSL(2,8), 504, 9 ][ PSL(2,11), 660, 8 ]
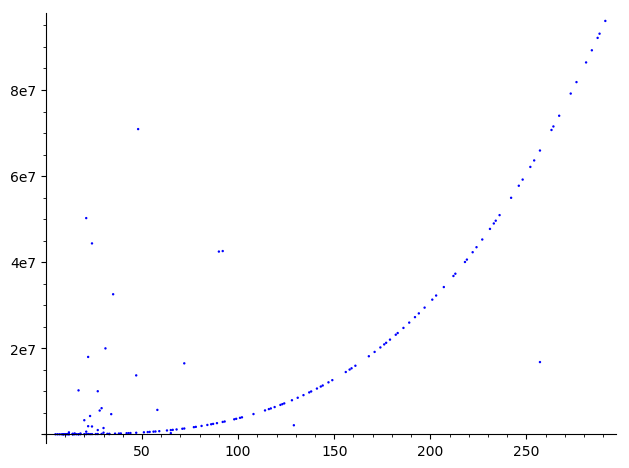
The points of the following picture corresponds to the $161$ finite simple groups of order less than $10^8$, where $x$-axis is the class number and $y$-axis the order.
We observe that these points are bounded below by the curve interpolating the points for the groups $\mathrm{PSL}(2,2^n)$. We checked that $k(\mathrm{PSL}(2,2^n)) = 2^n+1$, for $n \le 50$, so that the equality should be known true in general. Now $$|\mathrm{PSL(2,2^n)}| = 2^n (2^{2n}-1) = (r-1)((r-1)^2-1)) = (r-2)(r-1)r,$$ with $r = 2^n+1$, which leads to:
Question: Let $G$ be a finite simple group of class number $r$. Is it true that $|G| \ge (r-2)(r-1)r$?
If so, do you expect the existence of a proof without CFSG?
(because motivation: extend such a result to the simple integral fusion rings)
gap> for r in [5..15] do Print([r,(r-2)*(r-1)*r]); od;
[ 5, 60 ][ 6, 120 ][ 7, 210 ][ 8, 336 ][ 9, 504 ][ 10, 720 ][ 11, 990 ][ 12, 1320 ][ 13, 1716 ][ 14, 2184 ][ 15, 2730 ]
Bonus
We checked that $k(\mathrm{PSL}(2,3^n)) = (5 + 3^n) / 2$ for $n \le 40$ (see A289521), moreover, $$|\mathrm{PSL}(2,3^n)| = \frac{1}{2}3^n (3^{2n}-1) = \frac{1}{2}(2r-5)((2r-5)^2-1)) = \frac{1}{2}(2r-6)(2r-5)(2r-4),$$ with $r = \frac{1}{2}(5 + 3^n)$. These groups seems to be in the main curve in the middle. Then:
Augmented question: Let $G$ be a finite simple group of class number $r$. Is it true that $$|G| \ge (r-2)(r-1)r,$$ and that the equality holds iff $G = \mathrm{PSL}(2,2^n)$, otherwise that $G=\mathrm{PSU}(3,3)$ xor $$|G| \ge \frac{1}{2}(2r-6)(2r-5)(2r-4),$$ and that the equality holds iff $G = \mathrm{PSL}(2,q)$ with $q$ a odd prime power?
Remark: It is checked by GAP for $|G| < 15000000$.
gap> for r in [5..15] do Print([r,(2*r-6)*(2*r-5)*(2*r-4)/2]); od;
[ 5, 60 ][ 6, 168 ][ 7, 360 ][ 8, 660 ][ 9, 1092 ][ 10, 1680 ][ 11, 2448 ][ 12, 3420 ][ 13, 4620 ][ 14, 6072 ]
[ 15, 7800 ]