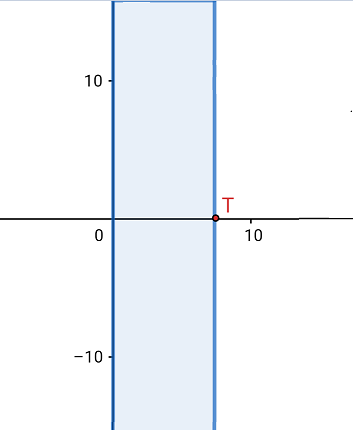
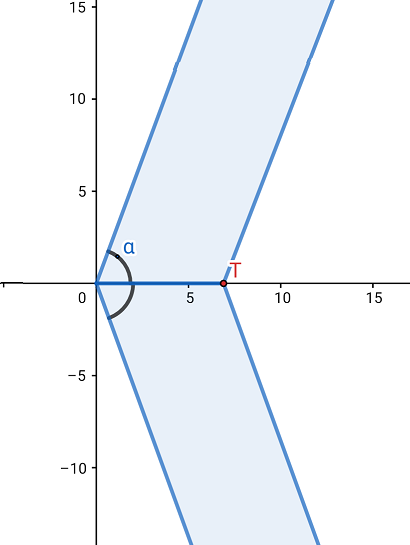
Consider the vertical strip of angle $\alpha=\frac{\pi}{2}$
In this case, the harmonic function which is $0$ on the left line and $1$ on the right line is given by $$f(a+ib)=\frac{a}{T}.$$ Now, when the angle $0<\alpha <\frac{\pi}{2}$ the strip becomes bent.
My question is: can we determine explicitly the harmonic function that is $0$ on left half lines and $1$ on the right ones. My attempt gave the following function $$f(a+ib)=\frac{a}{T}- \frac{\cos(\alpha)}{\sin(\alpha)}\frac{|b|}{T},$$ but this is not a harmonic function. Thank you for any hint.