The expression you've coined reflects the orbit of one initial number $s$ towards $1$ by (the Syracuse-notation of) the Collatz-transformation. A perhaps better expression for this is
$$ a_{N+1} = \small {3^N a_1 + (3^{N-1} + 3^{N-2} \cdot 2^{A_1} + 3^{N-3} \cdot 2^{A_1+A_2} + ... + 3 \cdot 2^{A_1+A_2+...+A_{N-2} } + 2^{A_1+A_2+...+A_{N-2}+A_{N-1} } )\over 2^{A_1+A_2+...+A_{N-2}+A_{N-1} + A_N } }
$$
where the denominator is your expression $2^m$ .
Let's make this monster-expression shorter; express the parenthese by the shortform $$ Q([A_1,A_2,...,A_N])=3^{N-1}+3^{N-2}\cdot 2^{A_1} + \cdots + 2^{A_1 +...+ A_{N-1}} $$ and $S = \sum_{k=1}^N A_k$ (I like the capital letters $N$(-umber-of-steps/-exponents) and $S$(-um-of-exponents) and $A_k>0$ for the terms in exponents instead of small letters like $m$ as you use it here).
Then we have in general
$$ a_{N+1} = a_1 \cdot {3^N \over 2^S} + {Q([A_1,...,A_N])\over 2^S} $$
Your first question is to prove, that for all odd $a_1$ there is a vector $E(a_1)=[A_1,A_2,...,A_N]$ such that $a_1 \mapsto 1$ by finitely many $N$ steps.
As it is well known, nobody has a proof so far and thus the Collatz-problem remains an open problem until now.
Your other observation is that of properties of three-step orbits ending at $1$. For this I propose to revert the notation: which numbers $a_3$ can be reached by the inverse Collatz-transformation of $N=3$ steps.
We can write it this way:
$$ a_{k-1} = {a_k 2^{A_k}-1\over 3} \qquad \text{where } a_k \equiv 2^{-A_k} \pmod 3$$
and rewriting
$$ a_{N+1} = a_1 \cdot {3^N \over 2^S} + {Q([A_1,...,A_N])\over 2^S} \\\
a_4 = a_1 \cdot {3^3 \over 2^S} + {Q([A_1,A_2,A_3])\over 2^S} \\\
1 = a_1 \cdot {3^3 \over 2^S} + {Q([A_1,A_2,A_3])\over 2^S} \\\
2^S = a_1 \cdot {3^3 } + {Q([A_1,A_2,A_3])} \\\
2^S - {Q([A_1,A_2,A_3])} = a_1 \cdot {3^3 } \\\
{2^S - Q([A_1,A_2,A_3]) \over 3^3} = a_1 \\\
$$
Of course, ${1\cdot 2^{A_3}-1\over 3}$ being integer means $A_3=2k_3$ is even, and given your demand that $a_4=1$ gives $a_3={4^{k_3}-1\over3} = \{1,5,21,85,...\}$
Along that line the possible values for $a_2$ and then for $a_1$ can be determined.
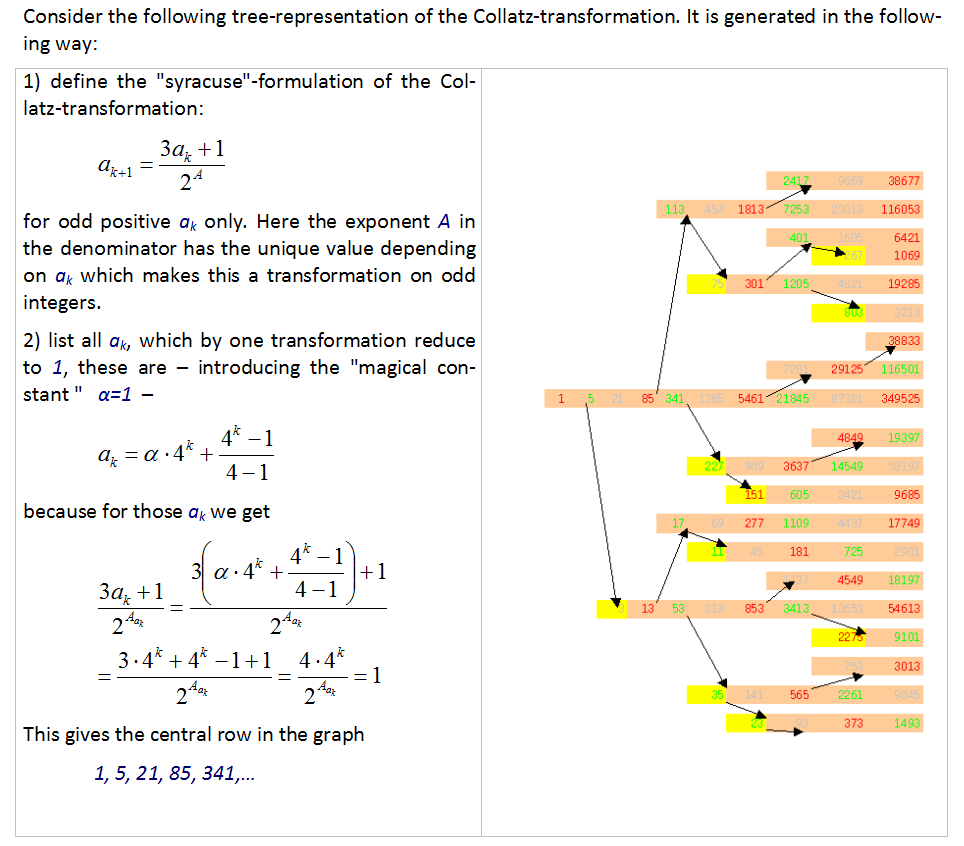
In an older webpage I've drawn a tree where you can identify the possible $a_1$ starting at $a_4=1$ applying $3$-times the reverse odd steps . The vector of $[A_1,A_2,A_3]$ here is surely identical to what you have found yourself, but, well, there's not much to prove here: just to determine the possible values due to simple modular conditions (such that for instance $A_3$ must be even).
The following picture is a graph excerpted from one manuscript. Numbers $a_k$ on one horizontal row transfer to the same number by one transformation. Reading the tree backwards (in direction of the arrows!) you can get an image, which numbers can be created by an inverse 3-step-transformation...