There is a refinement of the connect-sum decomposition called 'satellite operation' or 'splicing'. Like the connect-sum operation, it's originally due to Schubert, although Schubert didn't prove a uniqueness theorem. That came much later with the JSJ-decomposition.
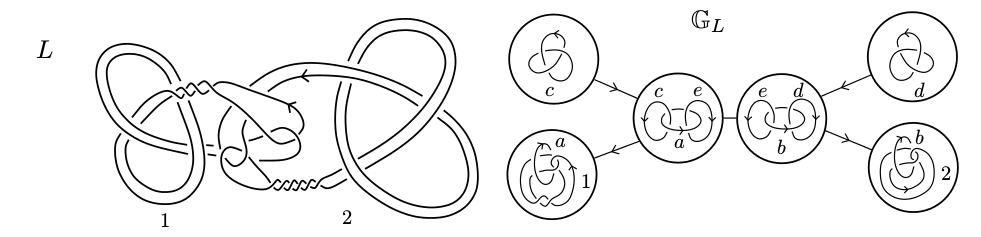
Unfortunately, this consists of infinitely-many operations. You could think of it as one operation that takes as input $n$ knots and an $(n+1)$-component link $L = (L_0, L_1, \cdots, L_n)$ such that the sublink $(L_1, \cdots, L_n)$ is trivial. You also demand that the complement of $L$ in $S^3$ is either Seifert-fibred or hyperbolic. It's also an essentially unique decomposition (like the connect-sum decomposition) -- the link is well defined but the indexing of the knots is only unique up to the symmetry group of the link $L$.

This is called the Whitehead double of a figure-8 knot. The link $W$ (in the $\mathbb G_K$ diagram) is the Whitehead link, the input knot (only one) is the figure-8 knot.
For these definitions there is a framing convention, twisted Whitehead doubles would decompose as:
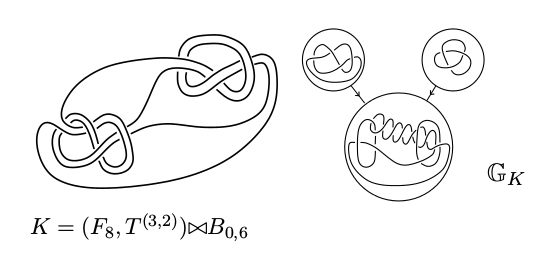

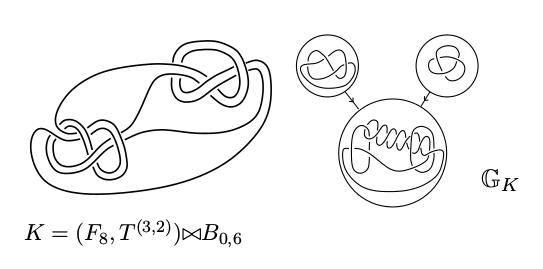
This doesn't have a standard name. I like to call it a "(twisted) Borromean double". The input link is the Borromean rings (technically, a twisted version of them), and the input knots are two trefoils. Another example:
This decomposition also applies to links in $S^3$. Here is a picture of the decomposition in an interesting case: