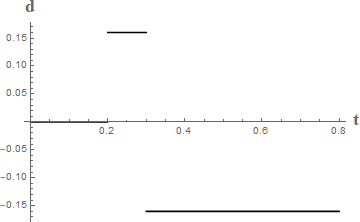
The answer is no. Indeed, suppose that $X_1,X_2,\dots$ are iid Bernoulli random variables (r.v.'s) each with mean $p$, $\bar X_n:=\frac1n\,\sum_1^n X_i$, and $$d_{n,p}(t):=P(|\bar X_{n+1}-p|>t)-P(|\bar X_n-p|>t). $$ The graph $\{\big(t,d_{1,1/5}(t)\big)\colon0\le t\le4/5\}$ is shown here:
We see that the (right-continuous) function $d_{1,1/5}$ takes values of both signs. In particular, $d_{1,1/5}(1/5)=4/25>0>-4/25=d_{1,1/5}(3/10)$.
Thus, the family of r.v.'s $(|\bar X_n-p|)_{n=1}^\infty$ is not stochastically monotone.