Yes, $K_{3,3}$ is such an example. $K_{3,3}$ is cubic, i.e., $3$-regular, but not planar.
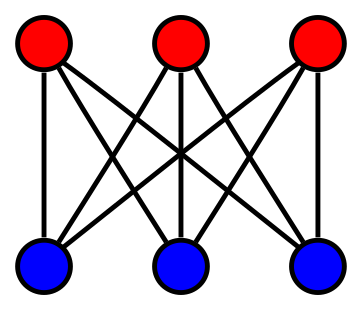
Image from [Wikipedia](https://en.wikipedia.org/wiki/Cubic_graph).
[Steinitz's theorem](https://en.wikipedia.org/wiki/Steinitz%27s_theorem) says that the polyhedral graphs are ($1$-skeletons of convex polyhedra) are exactly the $3$-connected planar graphs (with at least $4$ vertices).
