Let $\text{sPre}(C)$ be the category of simplicial presheaves with Grothendieck topology $\tau$. Let $\pi_0^{\tau}$$\pi_n^{\tau}$ be the $\tau$-homotopy sheaves for which $\pi_0^{\tau}(X)$ is the sheafification of the presheaf $U\mapsto [U,X]
$, where $[-,-]$ is the homotopy class of the $\tau$-local model category (the hom-set in the homotopy category).defined as follow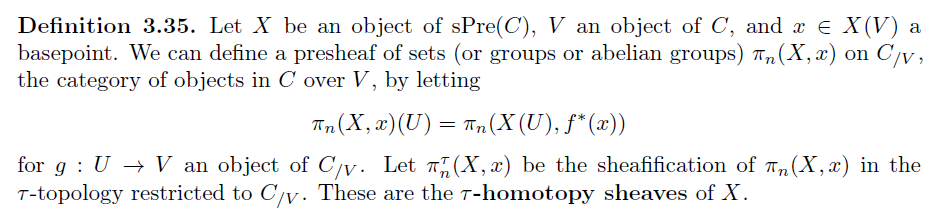
Does $\pi_0^{\tau}$$\pi_n^{\tau}$ commute with group completion $\Omega B(-)$? $\pi_0^{\tau}$$\pi_n^{\tau}$ commutes with $\Omega$ since sheafification functor is exact and the hom-functor commute with limits, but how about the classifying space functor $B$?
