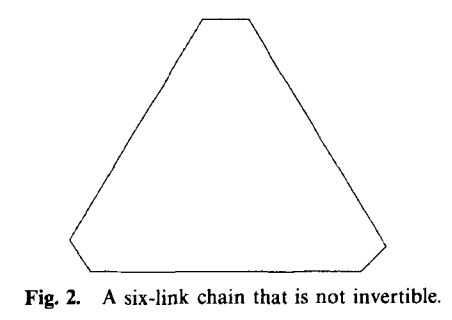
This question was explored here:
Lenhart, William J., and Sue H. Whitesides. "Reconfiguring closed polygonal chains in Euclidean $d$-space." Discrete & Computational Geometry 13, no. 1 (1995): 123-140; DOI: 10.1007/BF02574031, eudml.
From the Abstract: "It is shown that in three or more dimensions, reconfiguration is always possible, but that in dimension two this is not the case. Reconfiguration is shown to be always possible in two dimensions if and only if the sum of the lengths of the second and third longest links add to at most the sum of the lengths of the remaining links."
[![BillSue1][1]][1]