One recent development is the discovery of "zebra-like" colorings that avoid an equilateral triangle; before, it was unknown whether the only 2-coloring to avoid an equilateral triangle is the obvious alternating strip coloring. The relevant paper is http://arxiv.org/abs/math.CO/0701940.
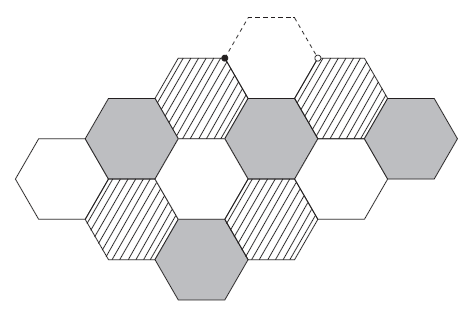
I have written on the subject. In http://www.math.ucsd.edu/~etressle/monotriangle.pdfhttp://nucularpower.com/papers/monotriangle.pdf I show a 3-coloring that avoids the 1,1,2 triangle. I will think about this for 2 colors. I believe it should be impossible, but I will update this later iftriangle; I have more thoughtsbeen unable to find a 2-coloring that does so. It is easy to see (due to Soifer) that either a 1,1,1 or a 2,2,2 triangle imply that a 1,1,2 triangle exists (for 2 colors, again). It is also known that no two-coloring simultaneously avoids equilateral triangles of sides 1, 2, and 3.
The general conjecture is still wide open, as is the conjecture that 3 colors suffice to avoid any given triangle.
Edit (1 August):Edit, December 2016: I have spent some time tryingupdated the link to prove that no 2my note. In case the link becomes outdated again, the 3-coloring that avoids the 1,1,2a degenerate triangle, and cannot prove it. If anyone makes progress on this, or knows the answer, or even is just wants to work on it with methe coloring shown below, please let me knowwhere the upper hexagon illustrates how boundaries are colored.