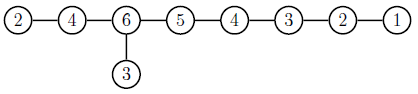
I don't believe there is a reference for this, for this follows immediately from the description of real roots in affine root systems. Namely, by Proposition 6.3(a) in "Infinite dimensional Lie algebras" by V. Kac the real roots of $\Delta=\mathsf{E}_8^{(1)}$ are of the following form. Number the simple roots as $\alpha_0,\alpha_1,\ldots,\alpha_8$, where $\mathring\Delta=\langle \alpha_1,\ldots,\alpha_8 \rangle$ is the subsystem of type $\mathsf{E}_8$, so $\alpha_0$ is the affine root. Then $$ \Delta^{\mathrm{re}} = \{ \alpha+n\delta \mid \alpha\in\mathring\Delta,\ n\in\mathbb{Z} \}, $$ where $\delta=\sum_{i=0}^8 c_i\alpha_i$, and the coefficients $c_i$ are as on the following diagram:
In what follows all roots are assumed to be real.
Now it's a matter of a simple calculation. The degree $0$ roots form a subsystem of type $\mathsf{A}_8$, so there are $36$ positive roots among them. The number of degree $1$, $2$ and $3$ roots in $\mathring\Delta$ is, respectively, $56$, $28$ and $8$. The degree $1$ roots in $\Delta$ are either in $\mathring\Delta$ or of the form $\alpha+\delta$, where $\alpha\in\mathring\Delta$ has degree $-2$, so there are $56+28=84$ of them; the same works for degree $2$ roots.
Degree $3$ roots are
- either in $\mathring\Delta$ (and form an $\mathsf{A}_7$ subsystem);
- or of the form $\alpha+\delta$, where $\alpha\in\mathring\Delta$ is of degree $0$;
- or of the form $\alpha+2\delta$, where $\alpha\in\mathring\Delta$ is of degree $-3$.
Thus in total there are $56+8+8=72$ roots of degree $3$.
Since there are no roots of degree $\geqslant3$ in $\mathsf{E}_8$, one concludes that $$ \#\left\{ \text{positive roots of degree $d$ in $\mathsf{E}_8^{(1)}$} \right\} = \begin{cases} 36, & \text{if}\ d=0, \\ 72, & \text{if}\ d \equiv 0 \pmod{3},\ d\neq 0, \\ 84 & \text{otherwise.} \end{cases}$$$$ \#\left\{ \text{positive real roots of degree $d$ in $\mathsf{E}_8^{(1)}$} \right\} = \begin{cases} 36, & \text{if}\ d=0, \\ 72, & \text{if}\ d \equiv 0 \pmod{3},\ d\neq 0, \\ 84 & \text{otherwise.} \end{cases}$$ The formula for the number of all real roots of a given degree (both positive and negative) is even more simple, namely, $72$ for $d\equiv0\pmod3$ and $84$ otherwise.