As mentioned by Gerald Edgar, you seek to simplify $$y = W(-r^{-1}xe^x)\Rightarrow x=W(-rye^y),$$ for $r>0$. Here is a series expansion in powers of $r$,
$$x=-\sum_{n=1}^\infty n^{n-1}\frac{1}{n!}(rye^y)^n.$$
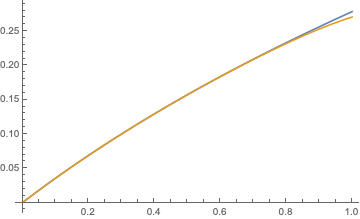
The plot compares the exact result for $x$ (blue curve) with the series expansion up to $n=5$$n=8$ (gold) at $y=1$$y=-1$, as a function of $r$. It's(I take $y<0$ because the OP says the interest is in $x>0$.) The series expansion remains quite accurate up toeven for $r=0.1$$r$ approaching unity.