EDIT I am terribly sorry, the question of course asked for a transfer map. I can leave my answer here if no one objects, but it is irrelevant for the question.
As it was already pointed out, there is a transfer map between the Galois groups induced by the class field theory isomorphisms. Another construction that I can think of comes from the isomorphism $K_0(R) \cong \mathbb{Z} \oplus \operatorname{Pic}(R)$ for every Dedekind domain $R$, and then one can apply the existance of transfers in algebraic $K$-theory.
Sorry again for the wrong answer.
I think that one can argue that $H_{k} \subseteq H_K$ and conclude that there is a restriction map between the Galois groups.
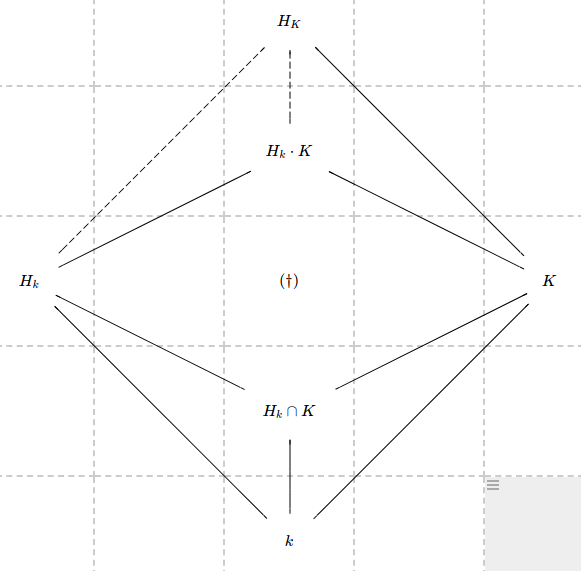
One way to do this is look at the diagram
and observe that $H_k \cdot K$ is unramified over $K$, because for every prime $\mathfrak{Q}$ of $H_k \cdot K$ we have that $$ e(\mathfrak{Q} \mid \mathfrak{P}) = e(\mathfrak{Q} \mid \mathfrak{p})/e(\mathfrak{P} \mid \mathfrak{p}) = e(\mathfrak{P'} \mid \mathfrak{p}) \cdot e(\mathfrak{Q} \mid \mathfrak{P}')/e(\mathfrak{P} \mid \mathfrak{p}) = e(\mathfrak{Q} \mid \mathfrak{P}')/e(\mathfrak{P} \mid \mathfrak{p}) = 1 $$ where $\mathfrak{P} := \mathfrak{Q} \cap K$, $\mathfrak{P}' := \mathfrak{Q} \cap H_k$ and $\mathfrak{p} := \mathfrak{Q} \cap (H_k \cap K)$. Observe that $e(\mathfrak{P'} \mid \mathfrak{p}) = 1$ because $H_k \cap K \subseteq H_k$ is unramified and $e(\mathfrak{Q} \mid \mathfrak{P}') = e(\mathfrak{P} \mid \mathfrak{p})$ as it follows from the following local argument.
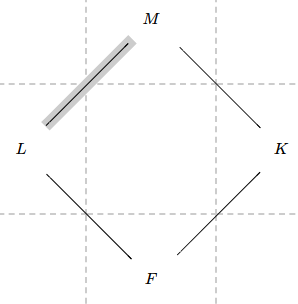
Consider a square of valued fields
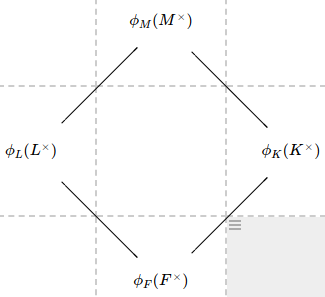
where $M = L \cdot K$ and $F = L \cap K$. Then it induces a diamond of groups
and thus $e(\phi_M \mid \phi_L) := [\phi_M(M^{\times}) \colon \phi_L(L^{\times})] = [\phi_K(K^{\times}) \colon \phi_F(F^{\times})] = e(\phi_K \mid \phi_F)$ as it follows from the second isomorphism theorem in group theory (see here, Theorem 10.3). Here of course, $\phi$ denotes the valuation associated to the given field.
Hence we can conclude that $H_K \supseteq H_k \cdot K$ by the characterizing property of the Hilbert class field, which is the biggest abelian extension of a field that is unramified everywhere. Hence $H_K \supseteq H_k$ and thus there is a restriction map $\operatorname{Gal}(H_K/K) \to \operatorname{Gal}(H_k/k)$.
On a side note, observe that this map could be trivial even if the two groups are not. An interesting example is when $H_k = K$ and $H_{H_k} \neq H_k$, which can happen even when $k$ is a quadratic field. In fact, it is a theorem of Golod and Shafarevic that if $\mathbb{Q} \subseteq k$ is an imaginary quadratic field ramified at more than six primes then it has an infinite class field tower (see this Harvard undergraduate thesis by Kathleen Zhou or this recent preprint by Farshid Hajir, Christian Maire and Ravi Ramakrishna).
P.S. Of course all along, $e(\cdot \mid \cdot)$ denotes the ramification index. For its definition in the context of valued fields see Section 3.3 of these notes by Peter Stevenhagen.