EDIT1:
In what follows I am pre-pending some omitted considerations regarding intersection of two small circles on a sphere resulting in two diangles, referring to them as the minor and major diangles or lunes.
At either end of two cutting planes the it makes $\alpha,\beta$ subtended between plane of small circles and the sphere's tangent plane.Angle $ \gamma$ is dihedral in between the planes.
The dihedral angle between the two planes is labelled with symbol $\gamma. $
Three dihedrals are taken in the tangent plane when considering three geodesic great circle arcs for a spherical triangle of three angles.
However it is not necessary here in lune/diangle situation. The boundaries need not be geodesics/great circles but can be small circles.
In the next edit I shall attempt extending this to three small circle triangle general spherical trigonometry.
The Cosine Rule in Spherical trig is equally valid here without geodesic boundaries, even when considering only small (const. latitude /parallel) circles
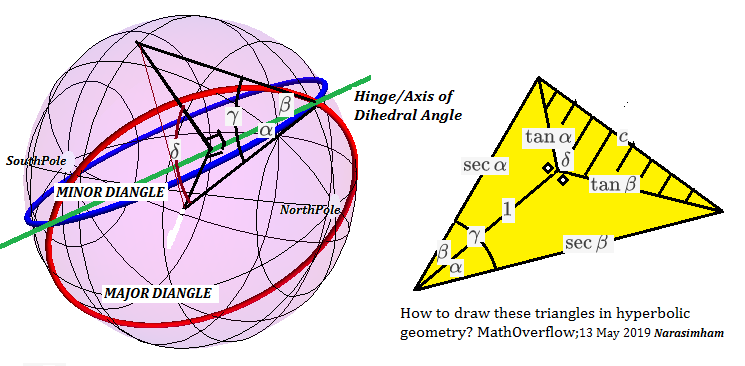
In the triangular pyramid shown we consider four triangles (two right triangles on a common hinge/fulcrum unit length normal to a striped triangle with a dihedral $\delta$ angle and the outer big yellow triangle containing compound angle $\gamma$).
We derive Cosine Rule in Spherical trigonometry indirectly avoiding representation of sphere radius.
By applying Cosine Rule in striped triangle
$$ c^2= \tan^2\alpha+\tan^2\beta-2\tan\alpha \tan\beta \cos \delta $$
By applying Cosine Rule in larger yellow triangle containing compound angle $\gamma$
$$c^2= \sec^2\alpha+\sec^2\beta-2\sec\alpha \sec\beta \cos \gamma$$
Eliminate $c^2$ to simplify we get Cosine Rule in spherical trigonometry
$$\cos \gamma= \cos\alpha\cos\beta+ \sin \alpha \sin \beta \cos \delta $$
We have used plane trig and embedded a pyramid into $\mathbb R ^3 $ without explicit reference to a sphere:
Now how can we draw the corresponding figure in hyperbolic geometry:
$$\cos \gamma= \cosh\alpha\cosh\beta+ \sinh \alpha \sinh \beta \cos \delta \,? $$
Considering simpler cases visualization... We can draw for right triangle $\delta= \pi/2$ the pyramid but how to at least draw it for hyperbolic geometry representation and result ?
$$ \cos \gamma= \cos\alpha\cos\beta \, \rightarrow \cos \gamma= \cosh\alpha\cosh\beta \,? $$
Thanks in advance for geometric considerations in hyperbolic geometry without explicitly bringing in the pseudosphere.
Regards