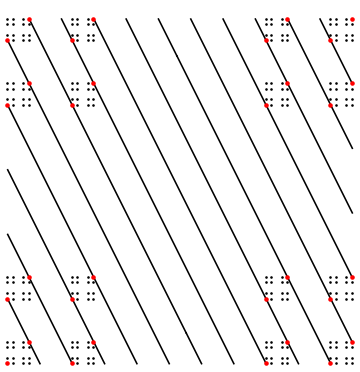
I decided to challenge myself to make pictures of Piotr Hajlasz's example, partly for fun and partly for the next time I teach this. Let $C_3$ be the standard Cantor middle thirds set: $$C_3 = \left\{ \sum_{k=1}^{\infty} \frac{b_k}{3^k} : b_1, b_2, b_3, \cdots, \in \{ 0,2 \} \right\}.$$$$C_3 = \left\{ \sum_{k=1}^{\infty} \frac{a_k}{3^k} : a_1, a_2, a_3, \cdots, \in \{ 0,2 \} \right\}.$$ Let $C_4$ be the variant "middle halves set" $$C_4 = \left\{ \sum_{k=1}^{\infty} \frac{b_k}{4^k} : b_1, b_2, b_3, \cdots, \in \{ 0,3 \} \right\}.$$ Note that every $z$ in $[0,1]$ can be written as $(2/3) x + (1/3) y$ for $x$, $y \in C_4$ in either $1$ or $2$ ways. Here is a drawing of $C_4 \times C_4$ and its intersection with the lines $(2/3)x + (1/3) y = k/16$ for $0 \leq k \leq 16$:
Here is a $C^1$ function $\phi(x)$ which maps $C_3$ to $C_4$ in an order preserving manner, with derivative $0$ at each point of $C_3$. (OnOn each interval of $[0,1] \setminus C_3$, it is an appropriately chosen sine curve.)
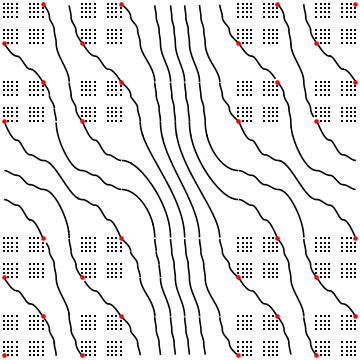
And here is the final product, a depiction of the function $f(x,y) = (2/3) \phi(x) + (1/3) \phi(y)$. The level curves show $f(x,y) = k/16$ for $0 \leq k \leq 16$. The black dots are the critical points $C_3 \times C_3$; the red dots demonstrate how every level curve contains $1$ or $2$ critical points.