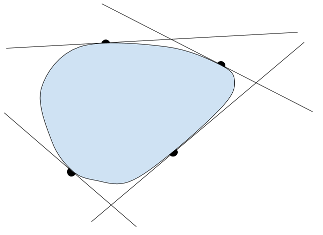
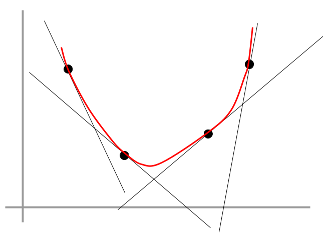
Apologies if this is basic -- I'm wondering where to find a proof and reference for the following facts, which I feel sure must be true.
(1) Suppose we are given a finite set of points in $\mathbb{R}^{d+1}$. For each point, we are given a closed halfspace tangent to it and strictly containing all others. Then there exists a strictly convex set contained in the intersection of the halfspaces with all the given points on the boundary.
(If I drop "strictly", then the convex hull of the points works.)
(2) Suppose we are given a finite set of pairs $(x,y)$ with $x \in \mathbb{R}^d$ and $y \in \mathbb{R}$. SupposeFor each point, we are given a linear function at each point tangent tothrough it and strictly below the others. Then there exists a strictly convex function passing through the points whose gradient at each given point matches the given linear function's.
(If I drop "strictly", then "convex closure" of the pairs works.)