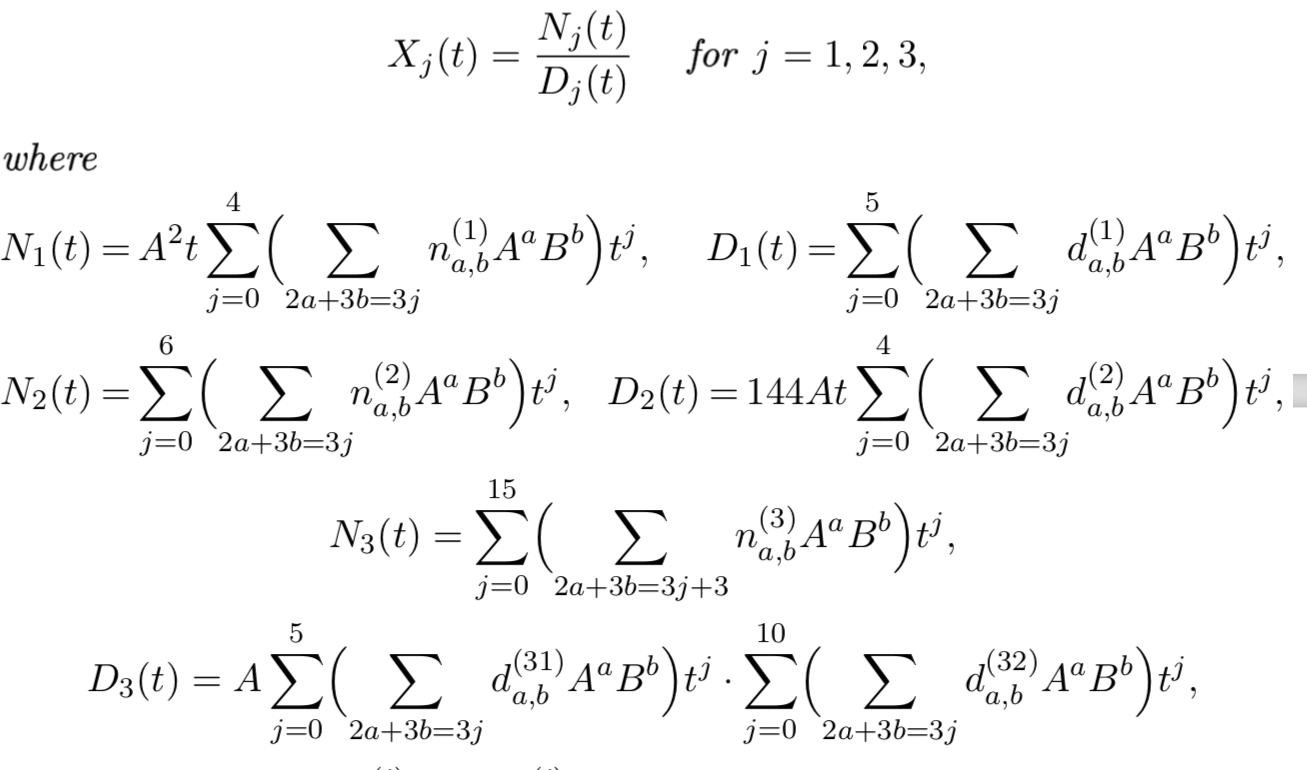Let $y^2 = x^3 + Ax + B$ be an elliptic curve over a field $F$ of characteristic not 2 or 3.

This paper of Skalba gives three degree 26 rational functions $X_1, X_2, X_3$ such that for any $t \in F$, exactly one of $X_1(t), X_2(t), X_3(t)$ represents the $x$ coordinate of a point on the curve. This is super useful for hashing into elliptic curves, which you sometimes need to do in cryptographic applications.

The rational functions are

where the $n_{a,b}, d_{a,b}$ are some constants.