While teaching a course in differential geometry, I came up with the following problem, which I think is cool.
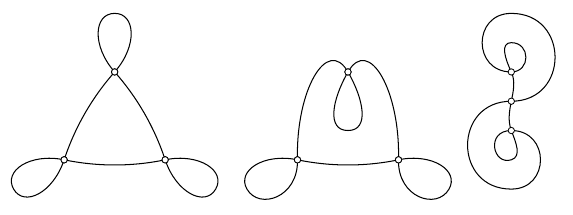
Assume $\gamma$ is a closed geodesic on a sphere $\Sigma$ with positive Gauss curvature. Can it look like one of the following curves in a parametrization of $\Sigma\backslash\{\text{point}\}$ by the plane?
I expect that there is a theorem that answers all questions like this. Is it indeed so?
$$ $$
P.S. The first curve can notcannot appear since by Gauss--Bonnet formula, the sum of angles in the triangle is $>\pi$. It makes the integral of Gauss curvature inside the loops $>4{\cdot}\pi$ which is impossible.
For theThe second curve I do not know thecannot appear as well, a proof is sketched in my answer. --- I guess(Please let me know if you see a way to simplify the answer is no, but it is not just Gauss--Bonnetproof.)
For the third one it is easy to produce an example --- say an ellipsoid has such geodesic.