Consider$\DeclareMathOperator\MCG{MCG}$Consider the mapping class group $MCG(\Sigma_2)$$\MCG(\Sigma_2)$ of the closed genus 2 oriented surface $\Sigma_2$. The algebraic-duality theory of $MCG_2:=MCG(\Sigma_2)$$\MCG_2:=\MCG(\Sigma_2)$ is explicitly described by Nathan Broaddus' very useful paper [https://arxiv.org/abs/0711.0011](arXiv link).
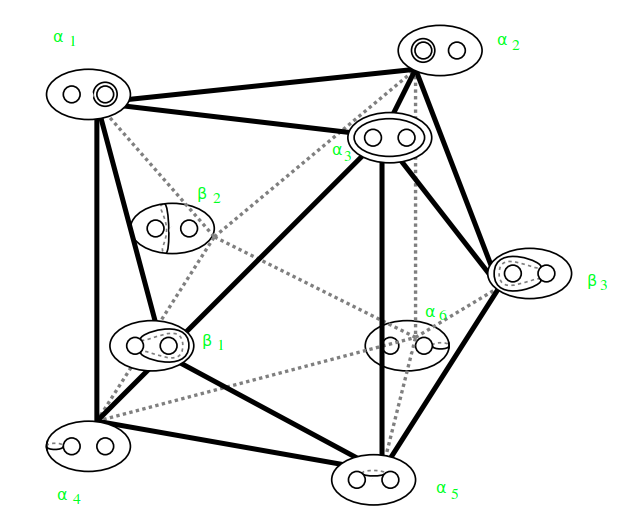
Broaddus constructs a beautiful homologically-nontrivial $2$-sphere $B$ in the curve complex of the genus two closed surface with marked curves α1, α2, α3, α4, α5, α6, β1, β2, β3 as labelled in the image below. ((we are indebted to N.Broaddus for his work and graphics.))
Question: I seek three distinct nontrivial elements $φ1,φ2,φ3$ of the mapping class group $MCG(\Sigma_2)$$\MCG(\Sigma_2)$ with the property that the following chain sum vanishes over $\mathbb{Z}/2$-coefficients:
$α1 + α2 + α3 + α4 + α5 + α6 + β1 + β2 + β3$$$α1 + α2 + α3 + α4 + α5 + α6 + β1 + β2 + β3$$
$+ α1.φ1 + α2.φ1 + α3.φ1 + α4.φ1 + α5.φ1 + α6.φ1 + β1.φ1 + β2.φ1 + β3.φ1$$$+ α1.φ1 + α2.φ1 + α3.φ1 + α4.φ1 + α5.φ1 + α6.φ1 + β1.φ1 + β2.φ1 + β3.φ1$$
$+ α1.φ2 + α2.φ2 + α3.φ2 + α4.φ2 + α5.φ2 + α6.φ2 + β1.φ2 + β2.φ2 + β3.φ2$$$+ α1.φ2 + α2.φ2 + α3.φ2 + α4.φ2 + α5.φ2 + α6.φ2 + β1.φ2 + β2.φ2 + β3.φ2$$
$+α1.φ3 + α2.φ3 + α3.φ3 + α4.φ3 + α5.φ3 + α6.φ3 + β1.φ3 + β2.φ3 + β3.φ3$$$+α1.φ3 + α2.φ3 + α3.φ3 + α4.φ3 + α5.φ3 + α6.φ3 + β1.φ3 + β2.φ3 + β3.φ3$$
By "vanishing over $\mathbb{Z}/2$-coefficients" we mean something utterly trivial like $\alpha+\beta + \alpha +\beta = 2 \alpha + 2\beta = 0$ (mod 2). Of course if we took $\phi_1 = \phi_2 =\phi_3=id$ or $\phi_1=\phi_2$, $\phi_3=id$ then the chain sum would vanish (mod 2).
Possibly a suitable finite subgroup $G'$ of $MCG_2$$\MCG_2$ will have the desired property. For the once-punctoredpunctured torus $\Sigma_{1,1}$, indeed the order 3 finite subgroup of $PGL(\mathbb{Z}^2)$ "closes" the necessary (Steinberg) symbol.
Believe it or not, but finding such a triple of elements would yield a $MCG$$\MCG$-equivariant codimension-two spine $Z \hookrightarrow Teich(\Sigma_2)$$Z \hookrightarrow \mathrm{Teich}(\Sigma_2)$ of the Teichmuller space.
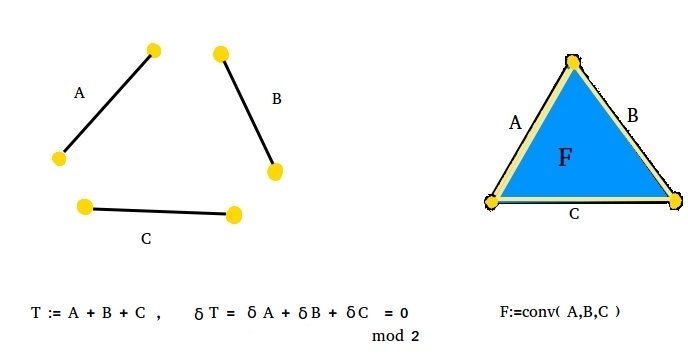
The problem is related to stitching footballs from uniform hexagonal panels, or uniform pentagonal panels, or combinations of both. To stitch a football from panels $\{P_i| i\in I\}$ means finding a finite subset $I' \subset I$ for which the singular chain sum $\sum_{i\in I'} P_i$ has singular chain boundary which vanishes mod $2$, so $$\partial(\sum_{i\in I'} P_i)=\sum_{i\in I'} \partial P_i=0$$ over $\mathbb{Z}/2$-coefficients. When $P$ is two-dimensional hexagon or pentagon, the panels have singular boundary $$\partial P= \sum_{e\text{~edge~of~}P} e.$$ We denote the closed convex hull of the football $F:=conv\{P|\text{~panels}\}$. The panels then become closed subsets of the boundary $\partial F$. For instance since the 1960's, the standard football is stitched after Adidas' ``Telstar" design, having twenty white hexagon panels, and twelve black pentagon panels. But in our applications we assume the patches $\{P_i\}_I$ are pairwise isometric to some regular geodesically-flat polygon $P$.