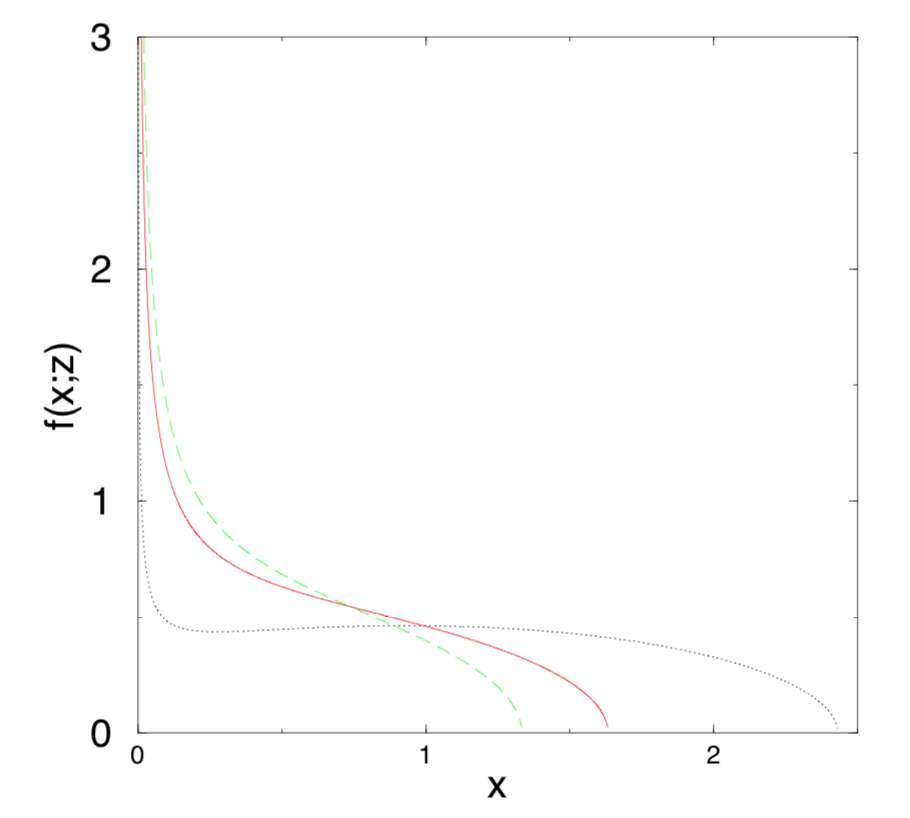
The average density of states in the Gaussian ensembles whose eigenvalues are restricted to lie in a given interval, or to be creatergreater than a given minimal value, was calculated by Dean and Majumdar in Extreme Value Statistics of Eigenvalues of Gaussian Random Matrices. The eigenvalue density has a $1/\sqrt x$ divergence at the end points of the interval. The case in the OP of a constraint of positive eigenvalues is the red solid curve in this plot from the cited paper.