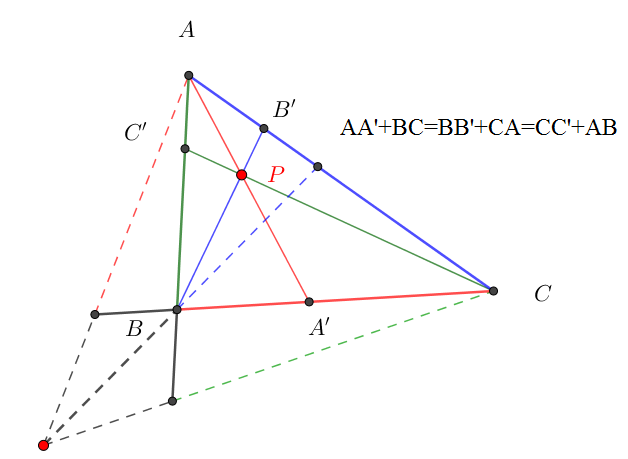
Let $ABC$ be a triangle and $P$ be a point on the plane, $PA$, $PB$, $PC$ meet $BC$, $CA$, $AB$ at $A'$, $B'$, $C'$ respectively.
From my construction by GeoGebra, I found two special points as follows:
In any triangle exist two points $P$ so that: $AA'+BC=BB'+CA=CC'+AB$.
My question: What is name of the points? Or are the points known?
RED=GREEN=BLUE