It seems that there are relations with $\mathrm{dis}(R)<\sqrt3$.
Take a regular heptagon $S_1\dots S_7$ inscribed into the unit circle $S$. Consider a partition into 7 parts $\mathcal P_1,\dots, \mathcal P_7$, and choose the points $A_1,\dots,A_7$ as shown below. Let $M_i$ be the point symmetric to $S_i$. All the numerations are cyclic modulo 7.
The correspondence is defined as follows.
$\bullet$ Every point in $\mathcal P_i$ corresponds to $S_i$.
$\bullet$ $A_i$ corresponds to each point of the arc $M_{i+3}M_{i+4}$.
![Partition][1]
[1]: https://i.sstatic.net/9Uf1I.jpg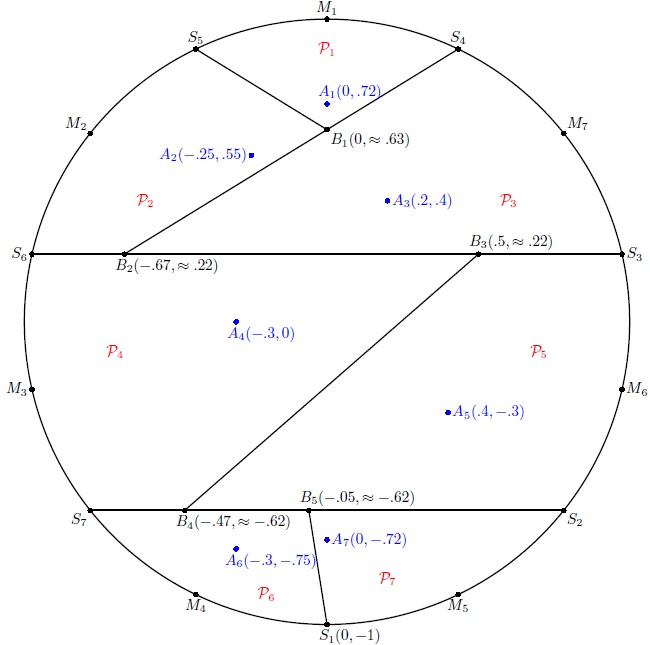
In order to show that $\mathrm{dis}(R)<d$ (with $d>S_1S_3$) for this relation, it suffices to do the following.
($\downarrow$) To show that $d(x,x')<d(y,y')+d$:
(1) $\mathrm{diam}(\mathcal P_i)<d$;
(2) $A_iA_{i+1}<d$;
(3) $H(A_i,\mathcal P_{i\pm 1})<d+S_1M_4=d+2\sin\frac\pi{14}$, where $H$ is the Hausdorff distance (i.e., the maximal distance from $A_i$ to a point in $\mathcal P_{i\pm 1}$; in fact, in our case this distance is even $<d$).
($\uparrow$) To show that $d(x,x')>d(y,y')-d$:
(4) $\rho(\mathcal P_i,\mathcal P_{i\pm 3})>2-d$;
(5) $A_iA_j>2-d$;
(6) $\rho(A_i,\mathcal P_{i\pm2})>S_1M_2-d=2\sin\frac{5\pi}{14}-d$.
One can easily check that all these conditions hold for $d=\sqrt3$.
DISCLAIMER. I did not try to optimize this example. Perhaps, it is possible to reach $\mathrm{dis}(R)<1.7$, or even $1.6$, in this way.
