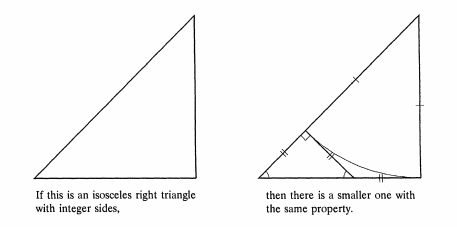
I wonder why Apostol's proof of the irrationality of $\sqrt{2}$ - which is as visual as a proof can be (in my opinion) - has not been mentioned: One can literally see at a glance that it proves what it's supposed to prove: the impossibility of a isosceles right triangle with integer side length (by infinite descent):
Note that it's not a proof completely without words. It helps a lot to read the comments of the author:
Each line segment in the diagram has integer length, and the three segments with double tick marks have equal lengths. (Two of them are tangents to the circle from the same point.) Therefore the smaller isosceles right triangle with hypotenuse on the horizontal base also has integer sides.
But through own thinking one could come up with this by oneself (having in mind what's to be proved).