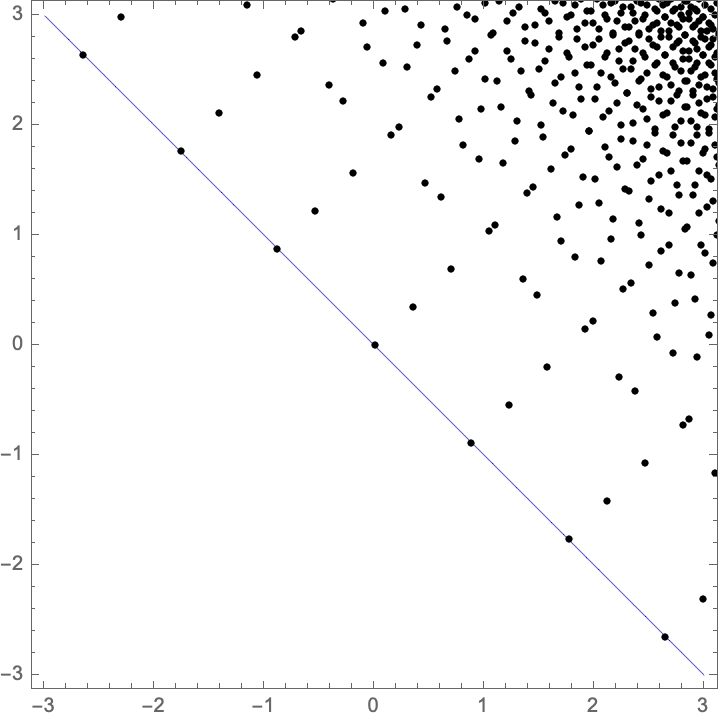
What I think of as the standard proof also gives a pretty clear picture. Let $K$ be a number field with $\rho_1$, $\rho_2$, ..., $\rho_r: K \to \mathbb{R}$ the real places and $\sigma_1$, $\sigma_2$, ..., $\sigma_s: K \to \mathbb{C}$ representatives with the complex places up to conjugacy. Define $\lambda: \mathcal{O}_K \to \mathbb{R}^{r+s}$ by $$\lambda(x) = (\log |\rho_1(x)|, \ldots, \log |\rho_r(x)|, 2 \log |\sigma_1(x)|, \ldots, 2 \log |\sigma_s(x)|).$$ Let $H \subset \mathbb{R}^{r+s}$ be the hyperplane where the sum of the coordinates equals $0$. Then $\lambda^{-1}(H)$ is the unit group of $\mathcal{O}_K$. The kernel of $\lambda$ is the roots of unity, and Dirichlet's unit theorem states that $\lambda(\mathcal{O}_K) \cap H$ is a lattice of rank $r+s-1 = \dim H$ in the hyperplane $H$.
Here is an image of $\lambda(\mathbb{Z}[\sqrt{2}])$, with the hyperplane $H$ in blue. (It's probably missing some pointsNotice that translation by a vector in $\lambda(\mathcal{O}_K) \cap H$ is a symmetry of the upper right; I restrictedimage; this corresponds to $a+b \sqrt{2}$ with $\max(|a|, |b|) \leq 10$multiplication by a unit.)