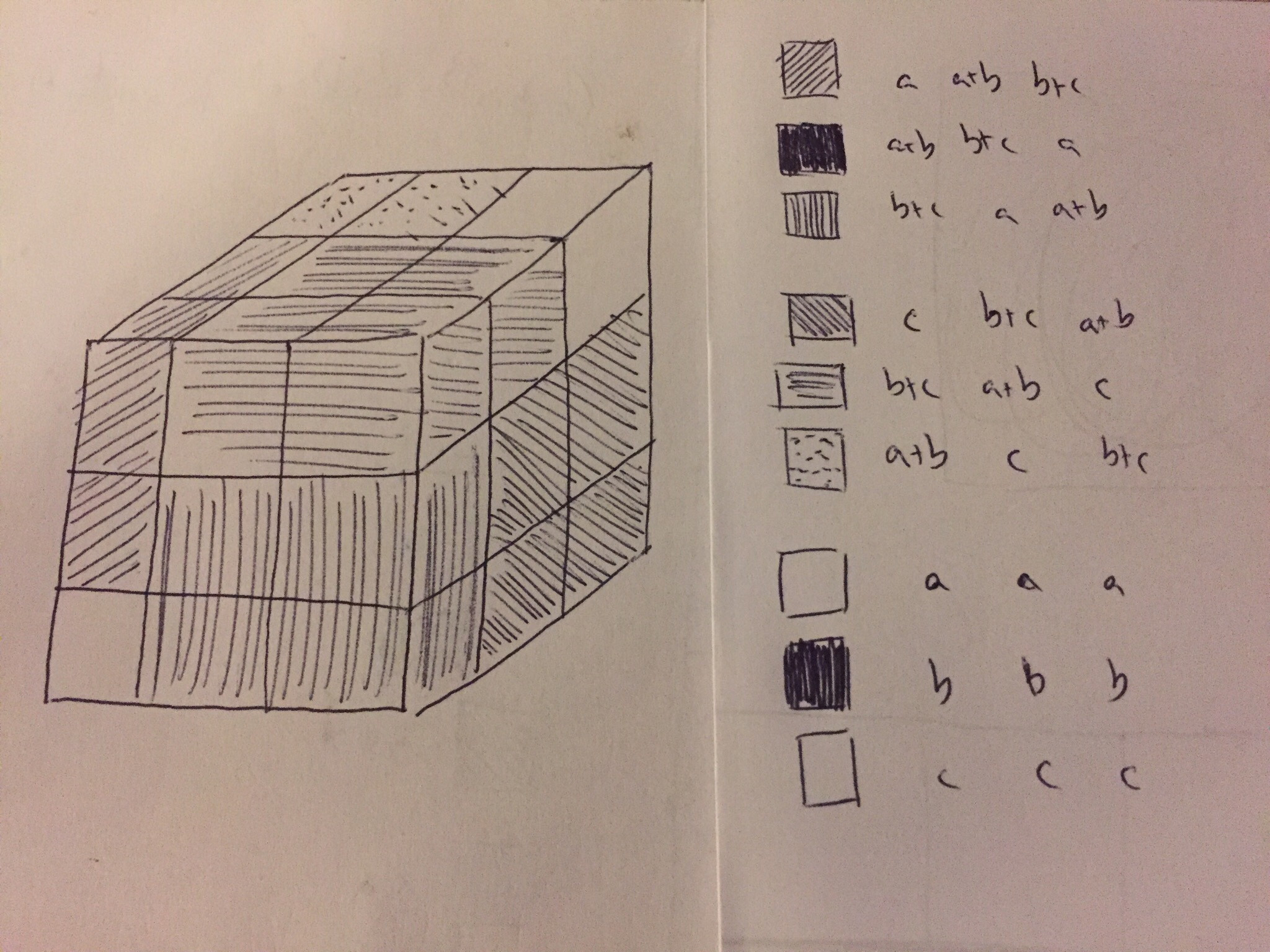
This shows the identity $$(a+b+c)^3 = a^3 + b^3 + c^3 + 3a(a+b)(b+c) + 3c(b+c)(a+b)$$ which builds on Jairo’s answer.
Each summand represents a block in the cube, ege.g. $a(a+b)(b+c)$ represents the block $(0,a) \times (0,b+c) \times (a,a+b+c)$$(0,a) \times (0,a+b) \times (a,a+b+c)$, and multiplication by 3 represents cyclic permutation through the axes. The cube is a sum of 9 smaller blocks, though two are not visible in the drawing.