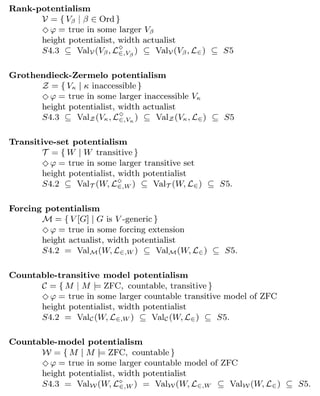
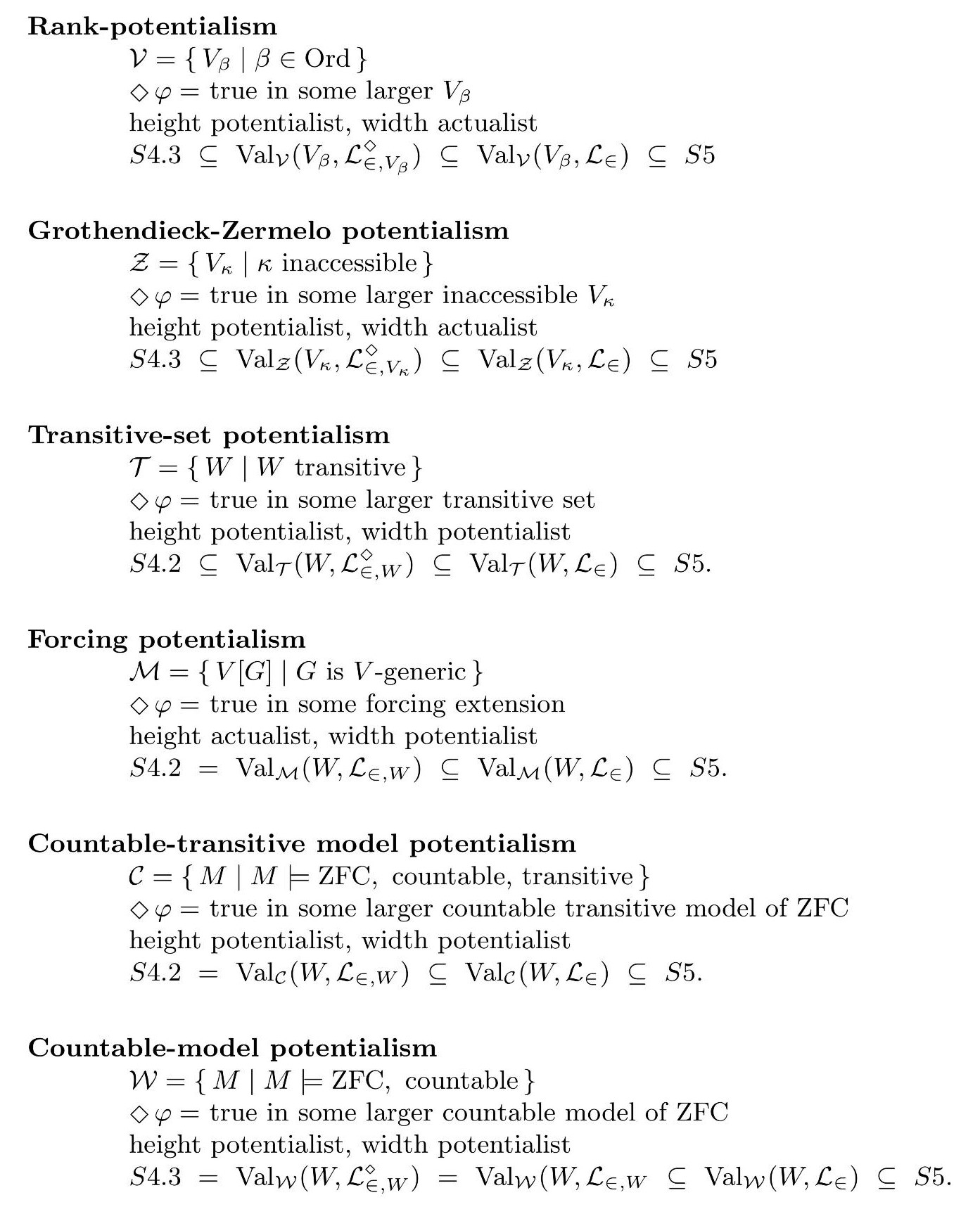
First, let us fix a countable model of ZFC plus V=L, say, and consider it in the context of all its outer models. Since we can force so as to destroy any stationary set in this model, while preserving others, we see that there is an infinite family of independent buttons, "the $n^{th}$ stationary set in the $L$-least partition of $\omega_1^L$ is no longer stationary." This can be made true in an outer model (by forcing) and once true, remains true in all further outer models; and the statements can be controlled independently. And since we also have a family of independent switches arising from the GCH patterns, it follows by the main modal logic analysis (as in Structural connections...), it follows that the modal logic of outer-models is contained within S4.2. And it certainly contains S4, because it is reflexive and transitive.