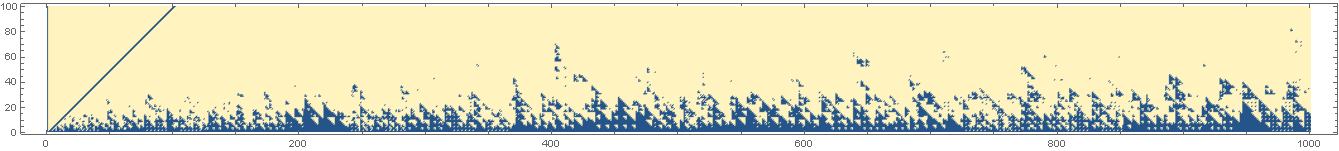
Just an illustration - for $m$ up to 6000, $r$ up to 120. Quite mysteriouslymysterious looking, I would say.
Version 2: in the coordinate system suggested by the answer of Lucia it looks somehow more regular, although, I believe, even more mysterious. Click if you want to enlarge.
This is now the plot of pairs $(n,r)$ with $\binom{n+r}n$ dividing $\binom{2n}n$.