From various considerations and with the help of J. Van der Jeugt, I was led to conjecture the following property of a class of Wigner 6j-symbols: for any integers $k,m$ with $m\ge k\ge 2$, $$ \left\{ \begin{array}{ccc} k & k & k \\ \frac{m}{2} & \frac{m}{2} & \frac{m}{2} \end{array} \right\}\ \neq\ 0 $$ except for the special case $k=2$, $m=3$.
Using Racah's single sum formula this amounts to the nonvanishing of the combinatorial sum $$ \sum_{n\in\mathbb{Z}} (-1)^n\ \left(\begin{array}{c} n+1 \\ 3k+1 \end{array}\right)\ \left(\begin{array}{c} k \\ n-k-m \end{array}\right)^3\ $$ with the usual convention that binomials are zero if the arguments are out of range.
Any ideas on how to prove this?
BTW, the exception $$ \left\{ \begin{array}{ccc} 2 & 2 & 2 \\ \frac{3}{2} & \frac{3}{2} & \frac{3}{2} \end{array} \right\}\ =\ 0 $$ has an interesting representation-theoretic interpretation (see this article by P. Minnaert).
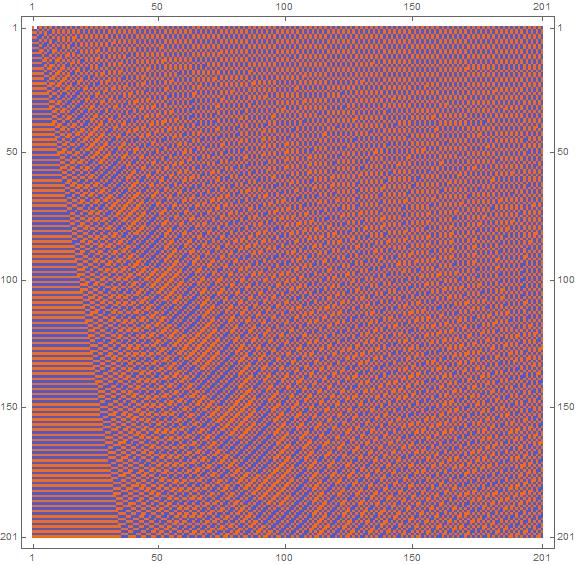
Also note that the signs of these symbols look quite complicated. Using a matrix plot on Mathematica for the output of
Table[Sign[SixJSymbol[{2+i, 2+i, 2+i}, {(2+i +j)/2, (2+i+j)/2, (2+i+j)/2}]],
{i, 0, 200}, {j, 0, 200}]
The exceptional zero mentioned above is the tiny white square in the top left corner.
Note that following Gerhard's suggestion of looking at the problem mod 3 one can rule out zeros away from the white spots in:
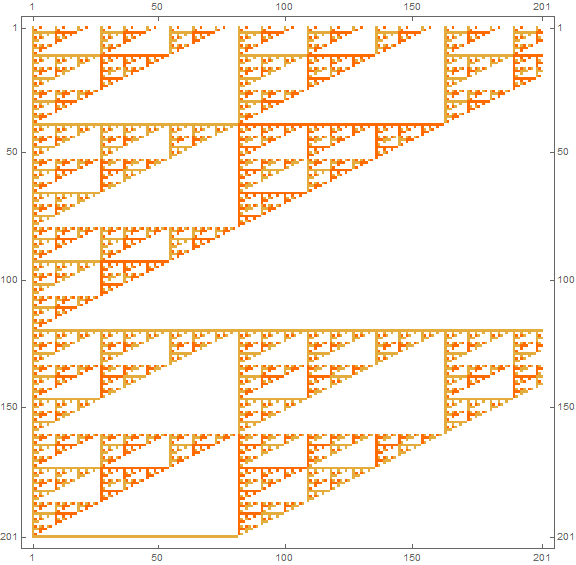
The above picture comes from using Fermat's Little Theorem to get rid of the third power mod 3 and the identity $$ \sum_{n\in\mathbb{Z}} (-1)^n\ \left(\begin{array}{c} n+1 \\ 3k+1 \end{array}\right)\ \left(\begin{array}{c} k \\ n-k-m \end{array}\right) \ =\ (-1)^m \left(\begin{array}{c} k+m+1 \\ 2k+1 \end{array}\right)\ . $$ This still leaves a piece of Swiss cheese.