Assuming the Riemann Hypothesis Montgomery consider the function $$F(\alpha)=F(\alpha,T)=\Bigl(\frac{T}{2\pi}\log T\Bigr)^{-1} \sum_{0<\gamma, \gamma'\le T} T^{i\alpha(\gamma-\gamma')}w(\gamma-\gamma')$$ where $w(x)=4/(4+x^2)$.
He proved results only in the range $-1+\varepsilon\le\alpha\le 1-\varepsilon$.
Therefore he restricted $\widehat r(\alpha)$ to this range and asserts
$$\sum_{0\le \gamma,\gamma'\le T} r\Bigl((\gamma-\gamma')\frac{\log T}{2\pi}\Bigr)
w(\gamma-\gamma')=\Bigl(\frac{T}{2\pi}\log T\Bigr)\int_{-\infty}^{+\infty}F(\alpha)\widehat{r}(\alpha).$$
From this result he obtains some consequences. For example, at least $2/3$ of the zeros are simple, that are proved conditionally on the Riemann hypothesis.
Then he give reasons to assume that $F(\alpha)\sim1$ for $|\alpha|\ge1$ and make his conjecture that $1-((\sin\pi u)/\pi u)^2$ is the pair correlation function for the zeros of the zeta function. This leads then to F. J. Dyson famous remarks.
So, you have to distinguish between what Montgomery proved under Riemann hypothesis and the conjecture of the correlation that goes beyond the Riemann hypothesis.
Montgomery's conjecture was reinforced by the statistics of Odlyzko. There is also another $\textbf{alternative hypothesis}$alternative hypothesis which postulates the normalized zeros tends to be separated by integers or half integers.
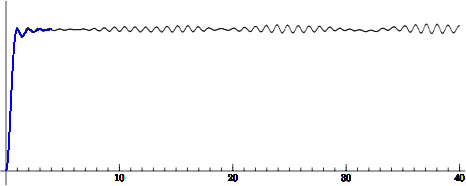
Odlyzko's figure was restricted to the range $0\le \gamma-\gamma'\le 6$. In 2013 I duplicated this statistics but using the range $0\le \gamma-\gamma'\le 40$. My figures revealed a surprising structure for this range, more in the mood of the alternative hypothesis.
I used Pratt zeros for this statistics, nevertheless it will be useful that somebody with more computer expertise than I cofirm my results. The figure is obtained from the zeros in the range 20.000.000 to 30.000.000 more or less.