I am looking for a proof of the problem as follows:
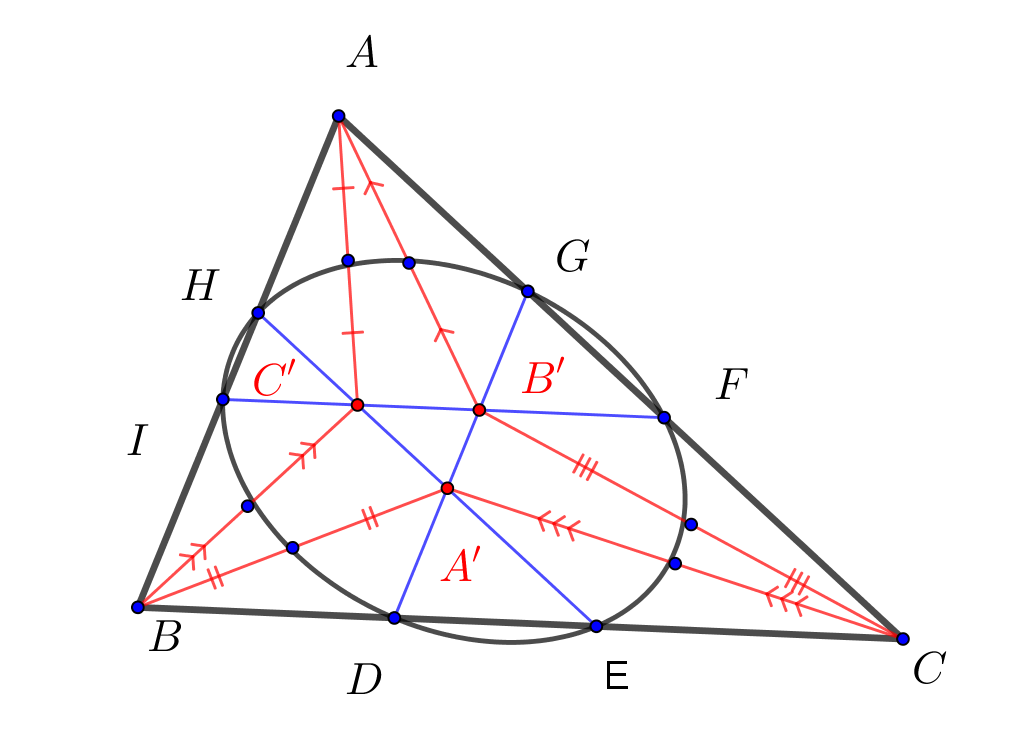
Let $ABC$ be a triangle, let points $D$, $E$ be chosen on $BC$, points $F$, $G$ be chosen on $CA$, points $H$, $I$ be chosen on $AB$, such that $IF$, $GD$, $EH$ parallel to $BC$, $CA$, $AB$ respectively. Denote $A'=DG \cap EH$, $B'=FI \cap GD$, $C'=HE \cap IF$, then 12 points:
$D$, $E$, $F$, $G$, $H$, $I$ and midpoints of $AB'$, $AC'$, $BC'$, $BA'$, $CA'$, $CB'$ lie on an ellipse if only if
$$\frac{\overline{BC}}{\overline{BE}}=\frac{\overline{CB}}{\overline{CD}}=\frac{\overline{CA}}{\overline{CG}}=\frac{\overline{AC}}{\overline{AF}}=\frac{\overline{AB}}{\overline{AI}}=\frac{\overline{BA}}{\overline{BH}}=\frac{\sqrt{5}+1}{2}$$
Note: This problem don't appear in AMM, and I don't have a solution for this problem, but there is the same configuration appear in:
UPDATE 07-Dec-2020:
Let $X$ is the centroid of $\triangle ABC$ and $X_1, X_2, X_3$ be three points lie on $XA, XB, XC$ such that:
$$\frac{XX_1}{XA}= \frac{XX_2}{XB}= \frac{XX_3}{XC}=\sqrt{2}\Phi^2$$
Where $\Phi=\frac{\sqrt{5}-1}{2}$
Amazingly, three points $X_1$, $X_2$, $X_3$ also lie on the Ellipse. So we should call the ellipse is: 15 Point Golden Ellipse.
