This answer is based on ideas quite different from those used in my previous answer to this question, and the result is much better. As in that answer, let \begin{equation*} S(x):=\sum_{k=0}^{\infty} \frac{x^k}{(k!)^2}. \end{equation*} Term-wise differentiation shows that \begin{equation*} (xS'(x))'=S(x). \end{equation*} This differential equation can be rewritten as \begin{equation*} 16x^2a''(x)+8x(1+4\sqrt x\,\tanh(2\sqrt x))a'(x)+a(x)=0, \tag{1} \end{equation*} where \begin{equation*} a(x):=S(x)/S_*(x), \end{equation*} \begin{equation*} S_*(x):=\cosh\sqrt{4x}/\sqrt{\pi\sqrt x}\sim e^{\sqrt{4x}}/\sqrt{4\pi\sqrt x}\sim S(x) \end{equation*} for large $x$, as was noted by Carlo Beenaker.

Note that $a(x)>0$; here and in what follows, $x>0$. So, by (1), $a'(x)=0$ implies $a''(x)<0$. So, the only local extrema of $a$ are local maxima; therefore and because between any two local maxima there is a local minimum, we see that there is at most one local maximum of $a$ on $(0,\infty)$. Since $a(0+)=0$, $a(1)>1$, and $a(\infty-)=1$, we conclude that $a$ has precisely one local (and hence global) maximum on $(0,\infty)$.

In fact, this maximum occurs at $x=x_*=0.7277\dots$, and $a(x_*)=1.0769\ldots<1.08$. Moreover, $a>1$ on $[0.2,\infty)$. Thus, \begin{equation} S_*<S<1.08\, S_*\quad\text{on }[0.2,\infty). \end{equation} These facts are illustrated by this graph of the function $a$:

$\newcommand{\de}{\delta} \newcommand{\De}{\Delta} \newcommand{\ep}{\epsilon} \newcommand{\ga}{\gamma} \newcommand{\Ga}{\Gamma} \newcommand{\la}{\lambda} \newcommand{\Si}{\Sigma} \renewcommand{\th}{\theta} \newcommand{\R}{\mathbb{R}} \newcommand{\F}{\mathcal{F}} \newcommand{\E}{\operatorname{\mathsf E}} \newcommand{\PP}{\operatorname{\mathsf P}} \newcommand{\ii}[1]{\operatorname{\mathsf I}\{#1\}}$

This answer is based on ideas quite different from those used in my previous answer to this question, and the result is much better. As in that answer, let \begin{equation*} S(x):=\sum_{k=0}^{\infty} \frac{x^k}{(k!)^2}. \end{equation*} Term-wise differentiation shows that \begin{equation*} (xS'(x))'=S(x). \end{equation*} This differential equation can be rewritten as \begin{equation*} 16x^2a''(x)+8x(1+4\sqrt x\,\tanh(2\sqrt x))a'(x)+a(x)=0, \tag{1} \end{equation*} where \begin{equation*} a(x):=S(x)/S_*(x), \end{equation*} \begin{equation*} S_*(x):=\cosh\sqrt{4x}\big/\sqrt{\pi\sqrt x}\sim e^{\sqrt{4x}}\big/\sqrt{4\pi\sqrt x}\sim S(x) \end{equation*} for large $x$, as was noted by Carlo Beenaker.

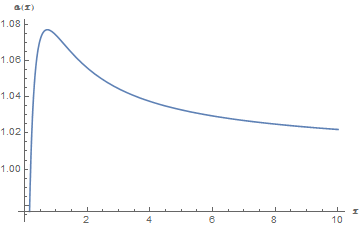
Note that $a(x)>0$ for $x>0$. So, by (1), if $a'(x)=0$ for some $x>0$, then $a''(x)<0$. So, the only local extrema of $a$ are local maxima; therefore and because between any two local maxima there is a local minimum, we see that there is at most one local maximum of $a$ on $(0,\infty)$. Since $a(0+)=0$, $a(1)>1$, and $a(\infty-)=1$, we conclude that $a$ has precisely one local (and hence global) maximum on $(0,\infty)$.

In fact, this maximum occurs at $x=x_*=0.7277\dots$, and $a(x_*)=1.0769\ldots<1.08$. Moreover, $a>1$ on $[0.2,\infty)$. Thus, \begin{equation*} S_*<S<1.08\, S_*\quad\text{on }[0.2,\infty). \end{equation*} These facts are illustrated by this graph of the function $a$:

To completely complete this answer, let us verify the mentioned asymptotic equivalence \begin{equation*} S(x)\sim\cosh\sqrt{4x}\big/\sqrt{\pi\sqrt x}; \tag{2} \end{equation*} here and in what follows, $x\to\infty$. Let $k_1,k_2,q_1,q_2$ be natural numbers such that \begin{align*} &k_1=x^{4/8}-\th x^{3/8},\quad q_1=x^{4/8}-\th x^{3/8}/2,\\ &k_2=x^{4/8}+\th x^{3/8},\quad q_2=x^{4/8}+\th x^{3/8}/2; \end{align*} here and elsewhere $\th$ denotes various expressions depending on $x$ (possibly different even in the same formula) such that $\th\to1$.
Write \begin{equation*} S(x)=S_1+S_2+S_3,\quad \cosh\sqrt{4x}=T_1+T_2+T_3, \end{equation*} where \begin{equation*} S_1:=\sum_{k=0}^{k_1-1} \frac{x^k}{(k!)^2},\quad S_2:=\sum_{k=k_1}^{k_2-1} \frac{x^k}{(k!)^2},\quad S_3:=\sum_{k=k_2}^\infty \frac{x^k}{(k!)^2}, \end{equation*} \begin{equation*} T_1:=\sum_{k=0}^{k_1-1} \frac{(4x)^k}{(2k)!},\quad T_2:=\sum_{k=k_1}^{k_2-1} \frac{(4x)^k}{(2k)!},\quad T_3:=\sum_{k=k_2}^\infty \frac{(4x)^k}{(2k)!}, \end{equation*}

Asymptotic equivalence (2) is an immediate consequence of the following two lemmas.

Lemma 1. $S_1+S_3<<S_2$ and $T_1+T_3<<T_2$; here and elsewhere $A<<B$ means $A/B\to0$.

Lemma 2. $S_2\sim T_2\big/\sqrt{\pi\sqrt x}$.

It remains to prove the lemmas.

Proof of Lemma 1. Let \begin{equation*} M:=M(x):=\max_{k\ge0}b_k,\quad b_k:=\frac{x^k}{(k!)^2},\quad r_k:=\frac{b_k}{b_{k-1}}=\frac{x}{k^2}. \end{equation*} Then $r_k$ is decreasing in $k$, $r_k\ge1$ for $k\le x^{4/8}$ and hence $b_k$ is nondecreasing in $k\le x^{4/8}$, $r_k\le1$ for $k\ge x^{4/8}$ and hence $b_k$ is nonincreasing in $k\ge x^{4/8}$. So, $M=b_{k_*}$ for some natural $k_*=k_*(x)=x^{4/8}+O(1)\in[k_1,k_2-1]$, and so, \begin{equation*} M\le \sum_{k=k_1}^{k_2-1}b_k=S_2. \end{equation*} Next, for $k\ge k_2$, \begin{equation*} b_k=b_{q_2}\prod_{j=q_2+1}^k r_j\le M r_{q_2}^{k-q_2}. \end{equation*} Also, \begin{equation*} r_{q_2}=\frac x{(x^{4/8}+\th x^{3/8}/2)^2}=(1+\th x^{-1/8}/2)^{-2}=1-\th x^{-1/8} \end{equation*} and $k_2-q_2=\th x^{3/8}/2$. So, \begin{multline*} S_3=\sum_{k=k_2}^\infty b_k\le M r_{q_2}^{k_2-q_2}\,\frac1{1-r_{q_2}} \sim M (1-\th x^{-1/8})^{\th x^{3/8}/2}\,x^{1/8} \\ =M \exp\{-\th x^{2/8}/2\}\,x^{1/8}<<M\le S_2. \tag{3} \end{multline*} Further, \begin{equation*} r_{k_1}=\frac x{(x^{4/8}-\th x^{3/8})^2}=(1-\th x^{-1/8})^{-2}=1+2\th x^{-1/8} \end{equation*} and $q_1-k_1=\th x^{3/8}/2$, whence \begin{multline*} b_{k_1}=b_{q_1}\Big/\prod_{j=k_1+1}^{q_1}r_j\le M/r_{k_1}^{q_1-k_1} =M/ (1+2\th x^{-1/8})^{\th x^{3/8}/2} =M /\exp\{\th x^{2/8}\} \end{multline*} and \begin{equation*} S_1=\sum_{k=0}^{k_1-1}b_k\le k_1 b_{k_1}\le x^{4/8}\,M /\exp\{\th x^{2/8}\}<<M\le S_2. \tag{4} \end{equation*} By (3) and (4), $S_1+S_3<<S_2$. That $T_1+T_3<<T_2$ is verified quite similarly; here instead of $r_k=\frac{x}{k^2}$, one will have to use $\frac{4x}{2k(2k-1)}=\frac{x}{k(k-1/2)}$. This completes the proof of Lemma 1. \qed

Proof of Lemma 2. For $k\in[k_1,k_2-1]$, we obviously have $k\sim\sqrt x$. So, using Stirling's formula, it is easy to see that for natural $k\in[k_1,k_2-1]$
\begin{equation*} \frac{x^k}{(k!)^2}\Big/\frac{(4x)^k}{(2k)!}=\frac{(2k)!}{(k!)^2}\frac1{4^k}\sim\frac1{\sqrt{\pi k}} \sim\frac1{\sqrt{\pi\sqrt x}}. \end{equation*} Now Lemma 2 immediately follows. \qed

This answer is based on ideas quite different from those used in my previous answer to this question, and the result is much better. As in that answer, let \begin{equation*} S(x):=\sum_{k=0}^{\infty} \frac{x^k}{(k!)^2}. \end{equation*} Term-wise differentiation shows that \begin{equation*} (xS'(x))'=S(x). \end{equation*} This differential equation can be rewritten as \begin{equation*} 16x^2a''(x)+8x(1+4\sqrt x\,\tanh(2\sqrt x))a'(x)+a(x)=0, \tag{1} \end{equation*} where \begin{equation*} a(x):=S(x)/S_*(x), \end{equation*} \begin{equation*} S_*(x):=\cosh\sqrt{4x}/\sqrt{\pi\sqrt x}\sim e^{\sqrt{4x}}/\sqrt{4\pi\sqrt x}\sim S(x) \end{equation*} for large $x$, as was noted by Carlo Beenaker.

Note that $a(x)>0$; here and in what follows, $x>0$. So, by (1), $a'(x)=0$ implies $a''(x)<0$. So, the only local extrema of $a$ are local maxima; therefore and because between any two local maxima there is a local minimum, we see that there is at most one local maximum of $a$ on $(0,\infty)$. Since $a(0+)=0$, $a(1)>1$, and $a(\infty-)=1$, we conclude that $a$ has precisely one local (and hence global) maximum on $(0,\infty)$.

In fact, this maximum occurs at $x=x_*=0.7277\dots$, and $a(x_*)=1.0769\ldots<1.08$. Moreover, $a>1$ on $[0.2,\infty)$. Thus, \begin{equation} S_*<S<1.08 S_*\quad\text{on }[0.2,\infty). \end{equation} These facts are illustrated by this picture:

This answer is based on ideas quite different from those used in my previous answer to this question, and the result is much better. As in that answer, let \begin{equation*} S(x):=\sum_{k=0}^{\infty} \frac{x^k}{(k!)^2}. \end{equation*} Term-wise differentiation shows that \begin{equation*} (xS'(x))'=S(x). \end{equation*} This differential equation can be rewritten as \begin{equation*} 16x^2a''(x)+8x(1+4\sqrt x\,\tanh(2\sqrt x))a'(x)+a(x)=0, \tag{1} \end{equation*} where \begin{equation*} a(x):=S(x)/S_*(x), \end{equation*} \begin{equation*} S_*(x):=\cosh\sqrt{4x}/\sqrt{\pi\sqrt x}\sim e^{\sqrt{4x}}/\sqrt{4\pi\sqrt x}\sim S(x) \end{equation*} for large $x$, as was noted by Carlo Beenaker.

Note that $a(x)>0$; here and in what follows, $x>0$. So, by (1), $a'(x)=0$ implies $a''(x)<0$. So, the only local extrema of $a$ are local maxima; therefore and because between any two local maxima there is a local minimum, we see that there is at most one local maximum of $a$ on $(0,\infty)$. Since $a(0+)=0$, $a(1)>1$, and $a(\infty-)=1$, we conclude that $a$ has precisely one local (and hence global) maximum on $(0,\infty)$.

In fact, this maximum occurs at $x=x_*=0.7277\dots$, and $a(x_*)=1.0769\ldots<1.08$. Moreover, $a>1$ on $[0.2,\infty)$. Thus, \begin{equation} S_*<S<1.08\, S_*\quad\text{on }[0.2,\infty). \end{equation} These facts are illustrated by this graph of the function $a$: