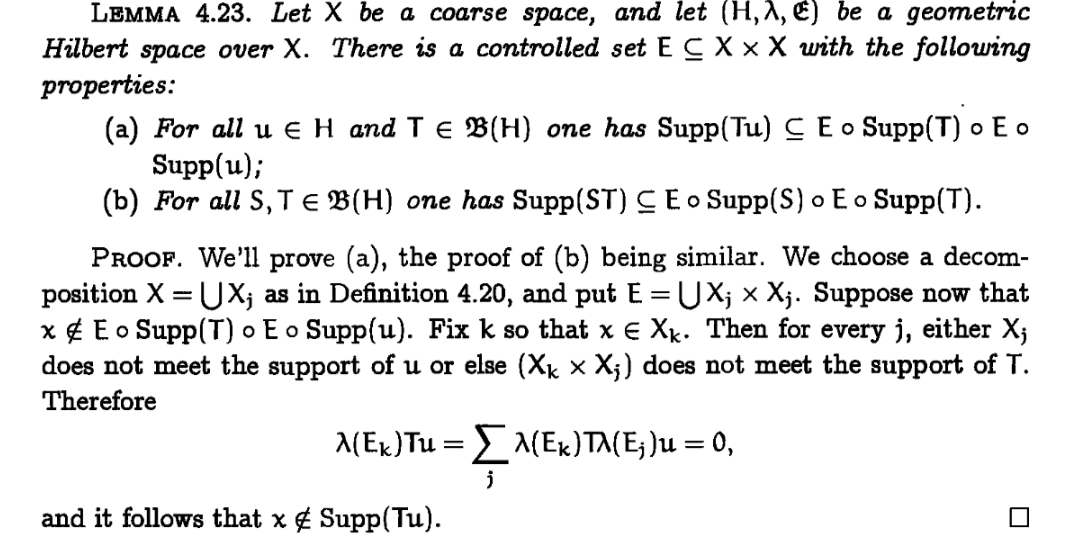The following picture is lemma 4.23 in Lectures on Coarse Geometry by John Roe:
I guess the $E_i$ in the centered formula is $X_i$. Does Roe mean that $X_j\cap \mathrm{Supp}(u)=\emptyset $ implies $\lambda(E_j)u=0$? But I can't work out. Can someone help me? (If more details such as definitions are needed, let me know.)