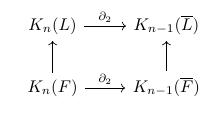Consider the Milnor $K_n$-functors for discrete valuiation fields. For any discrete valuation field $F$ we can associate an abelian group $K_n(F)$ and the construction is given thanks a universal property involving Steinberg map. We also have a map called $r$-th boundary map, which is given by: $$\partial_n: K_n(F)\to K_{n-1}(\overline F)$$

We can see $\partial_n$ as a transformation between functors, and my question is the following one:

Is $\partial_n$ a natural tranformation?

Suppose that we have an embedding of discrete valuation fields $F\to L$ which gives $\overline F\to \overline L$; then is the following diagram commutative?