This clarifies the notion of bundle of groups on $X$ with assumption that this is just another name for group bundle on $X$. Following screenshots are from book A general theory of fiber spaces with structure sheaf by Alexander Grothendieck. I thought it would be more authentic if I put screenshots than type it.

I have found the notion of group bundle in Alexandre Grothendieck's "A general theory of fibre spaces with structure sheaf".

This is for my own reference and may be useful for some one who reads that article Notes on $1$- and $2$-gerbes.

We need some definitions to go to the definition of group bundle and action $G\times _XP\rightarrow P$.

Definition : A fibre space over a space $X$ is a triple $(X,E,p)$ of the space $X$, a space $E$ and a continuous map $p$ of $E$ into $X$. A homomorphism of a fibre space $(X,E,p)$ to another fibre space $(X',E',p')$ is a pair of maps $f:X\rightarrow X'$ and $g:E\rightarrow E'$ such that $p'\circ g=f\circ p$. In this case $g$ maps fibres to fibres.

Definition : Let $(X,E,p)$ be a fibre space over $X$. Let $f:X'\rightarrow X$ be a continuous map. Then we define inverse image of fibre space $(X,E,p)$ to be the fibre space $(X',E',p')$ where $E'=\{(a,e):f(a)=p(e)\}\subseteq X'\times E$ and $p':E'\rightarrow X'$ is given by $p'(a,e)=a$.

Definition : Let $(X,E,p)$ be a fibre space and $(X,E',p')$ be another fibre space. This gives a map $$p\times p': E\times E'\rightarrow X\times X$$ with $(a,b)\mapsto (p(a),p'(b))$ making $(X\times X, E\times E', p\times p')$ into a fibre space. Consider diagonal map $\Delta:X\rightarrow X\times X$ given by $x\mapsto (x,x)$. Inverse image of $(X\times X, E\times E', p\times p')$ under $\Delta$ is what is called as fibre product of $(X,E,p)$ with $(X,E',p')$, denoted by $E\times_X E'$.

Definition : Let $E$ be a fibre space over $X$, provided with the supplement structure defined by a homomorphism of the fibre product $E\times_X E\rightarrow E$, or what is the same, a law of composition defined in each fibre $E_x$ such that the corresponding global map $E\times_X E\rightarrow E$ be continuous. This is called fibre space with composition law.

Definition : A group bundle $E$ over $X$ is a fibre space with composition law over $X$ such that for each $x\in X$, the fibre $E_x$ of $E$ is a group, the unit of which depends continuously on $x$, and that the map of $E$ into itself which on each fibre $E_x$ reduces to $z\mapsto z^{-1}$ be continuous.

Definition : Let $G$ be a group bundle on $X$ and $A$ be a fibre space on $X$. We say that $G$ operates at left on $A$ if we are given a homomorphism $G\times_X A\rightarrow A$ such that for each $x\in X$ the corresponding map $G_x\times A_x\rightarrow A_x$ is a group action. 

I am almost sure that by the unit of which depends continuously on $x$ it means the map $X\rightarrow E$ given by $x\mapsto e_x\in E_x\subseteq E$ is a continuous map, correct me if I am wrong.

In case of that article, $G$ is a bundle of groups on $X$ (i.e., fibre space $G\rightarrow X$) and $P\rightarrow X$ is a fibre space. Then there is fibre prodcut $(G\times_X P,X)$.

By $G\times_X P\rightarrow P$ it actually means homomorphism of fibre space $(G\times_X P,X)$ to $(P,X)$ i.e., it maps fibres to fibres in particular 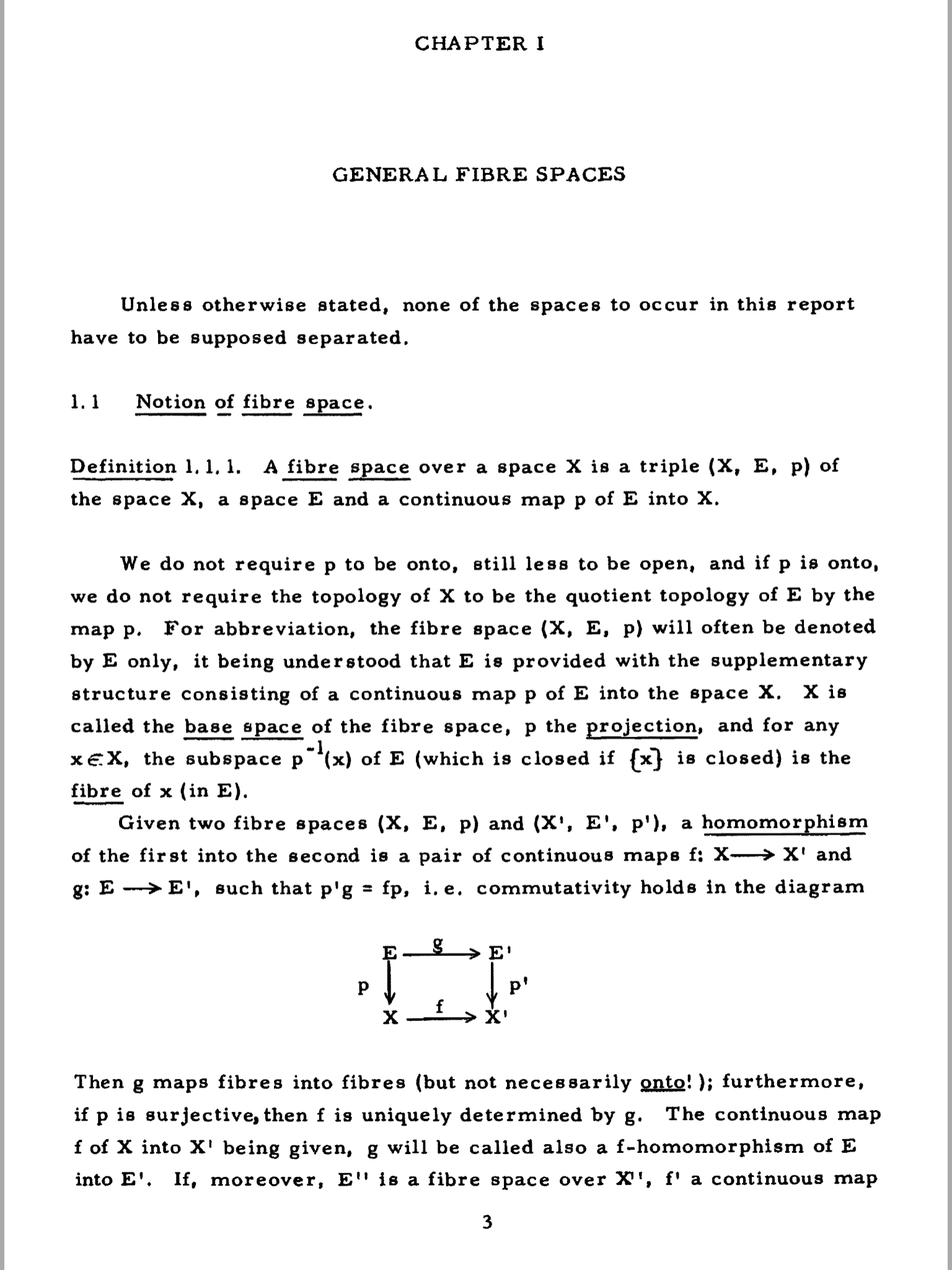$G_x\times P_x$ is mapped to $P_x$.