This answer gives some insight on eventually positivenonnegative matrices, which differs from the original question regarding power nonnegative matrices.
For results on power positive matrices, see Brauer [Duke Math. J. 28 1961 439–445; MR0130262]; for results on nonreal power nonnegative matrices see Tudisco et al. [Linear Algebra Appl. 471 (2015), 449–468; MR3314347].
The situation for the former is well-known for eventually positive matrices. Let $\rho(A)$ denote the spectral radius of $A$. Handelman [J. Operator Theory 6 (1981), no. 1, 55–74; MR0637001], Noutsos [Linear Algebra Appl. 412 (2006), no. 2-3, 132–153; MR2182957], and Johnson and Tarazaga [Positivity 8 (2004), no. 4, 327–338; MR2117663] showed that a real matrix $A$ is eventually positive if and only if $\rho(A)$ is a positive simple eigenvalue satisfying $$ |\lambda| < \rho(A) $$ for every $\lambda \in \sigma(A)$, and there are positive left and right eigenvectors $u$ and $v$ corresponding to $\rho(A)$ (this is known as the strong Perron Frobenius property).
It is also known that power index of $A$, which is the smallest positive integer $q$ such that $A^k$ is positive for all $k \ge q$ can be arbitrarily large. This is because, under very mild assumptions, arbitrarily large roots of eventually positive matrices remain eventually positive (see McDonald et al. [Matrix roots of eventually positive matrices. Linear Algebra Appl. 456 (2014), 122–137; MR3223894]). 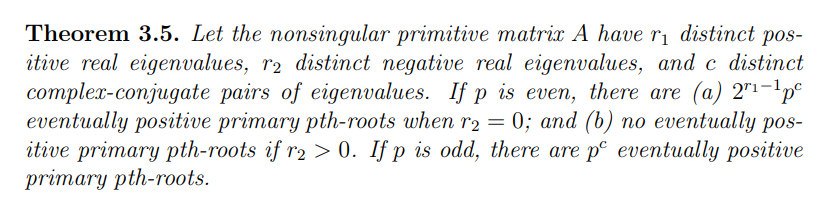
Another important work on eventually nonnegative matrices is by McDonald and Zaslavsky [A characterization of Jordan canonical forms which are similar to eventually nonnegative matrices with the properties of nonnegative matrices. Linear Algebra Appl. 372 (2003), 253–285; MR1999150].
It is, however, known that if $A$ is a primitive matrix, then $n^2 - 2n+2$ is a sharp upper bound on the index of primitivity (see Chapter 8 of Matrix Analysis by Horn & Johnson).
