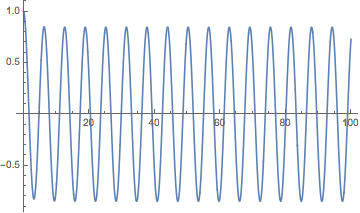
The large-$x$ limit is only zero if $a+b>3/2$: $$_1{F}_2({1}; {a, b}; -x^2/4)=$$ $$=\frac{2^{a+b}\Gamma (a) \Gamma (b)}{4\sqrt\pi} \left[\sin \left(\tfrac{1}{2} \pi (a+b)-x\right)-\cos \left(\tfrac{1}{2} \pi (a+b)-x\right)\right]x^{-a-b+3/2}+{\cal O}(1/x^2)$$$$=\sqrt{\tfrac{1}{\pi}}\Gamma (a) \Gamma (b) \sin \left(\tfrac{\pi}{2} (a+b-\tfrac{1}{2})-x\right)(2/x)^{a+b-3/2}+{\cal O}(1/x^2)$$
For example, when $a=b=1/2$ the amplitude of the oscillations increases as $\sqrt x$ (left plot), and when $a=b=3/4$ the amplitude does not decay (right plot):