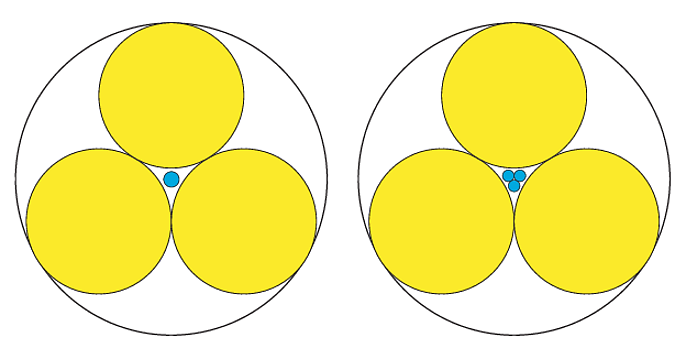
Here are examples of balanced circle packings whose touching graphs are disconnected:
Circles of same color are congruent and of equal weight. The drawing on the right shows how recursion can produce an arbitrarily large number of connected components. The very same number of circles and with the same arrangement pattern works in every dimension $d\ge2$; just replace each circle, including the container, with a concentric $d$-dimensional ball of the same radius.
Another example: $D_1$ and $D_2$ touch the boundary of $D$ and each other, but $D_i$ (for $i>2$) touches neither the boundary of $D$ nor any of the other disks.