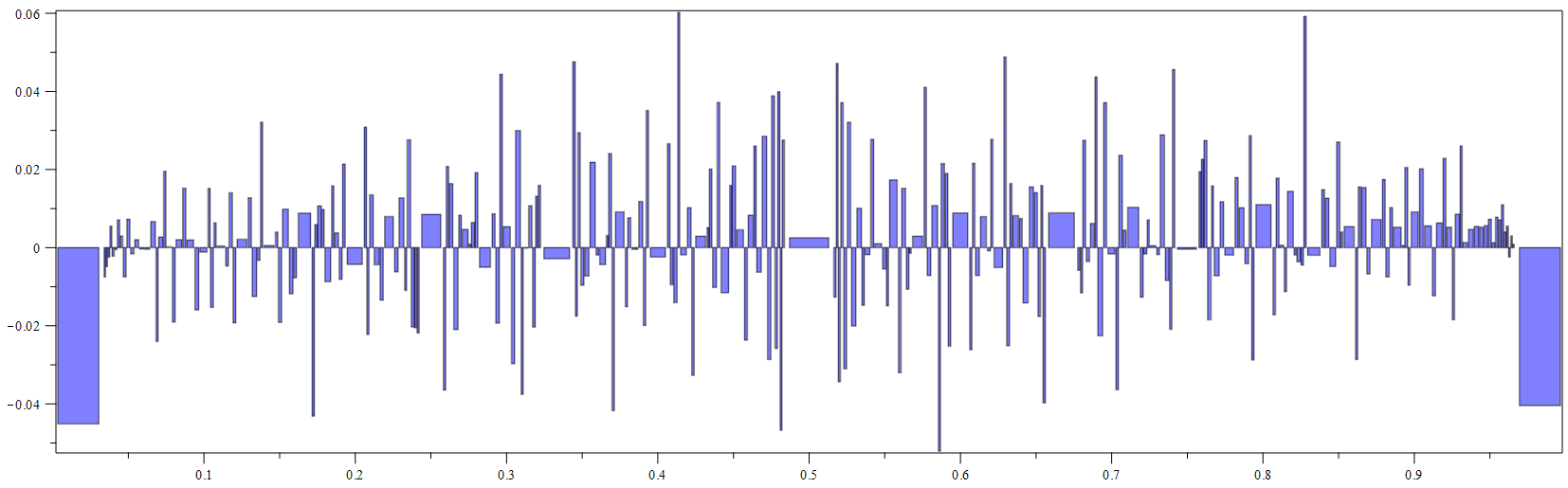
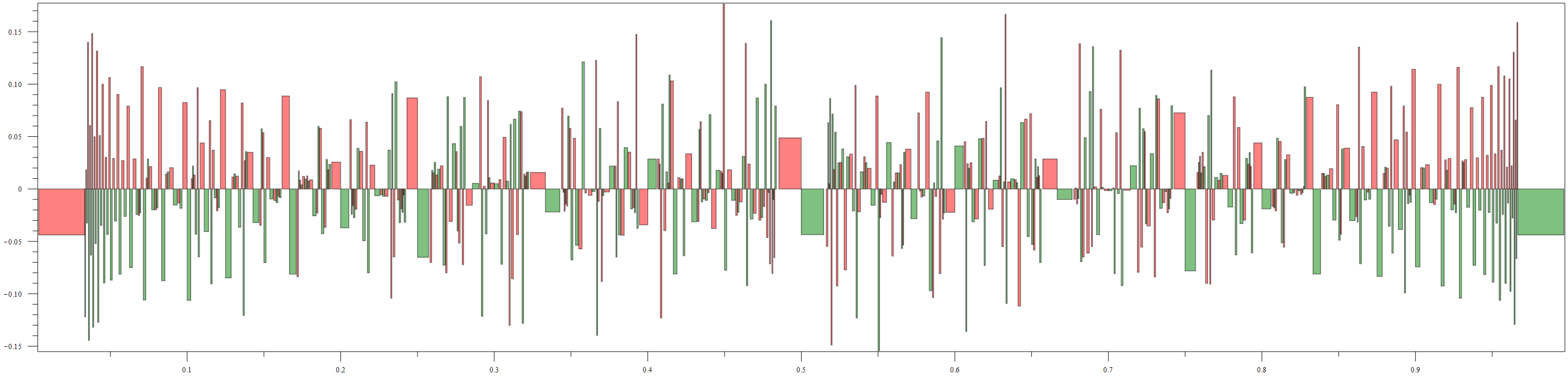
Farey Bins
For a rational number $\frac{a}b$ and integer $n$ we have $|\sqrt{n}-\frac{a}b|$ no smaller than about $\frac2{\sqrt{n}b^2}.$ This pushes (the fractional part of) square roots away from rational numbers with a force which is relatively stronger when the denominator is smaller. This is why if one wants the fractional part of $\sqrt(n)$ to be within $\frac{1}{1000}$ of $\frac12$ one needs $\sqrt{n}$ over $125.$ In fact $\sqrt(125^2+125+1)=125.5-0.000996$ and to be within $\frac{1}{1000}$ from above turns out to require $sqrt{n}$ over $375.$
If one wants to bin to study this I would suggest taking the small denominator fractions as roughly the centers of bins. Below are two graphs illustrating this. I suggest using mediants as the bin boundaries. For example, sorting the frasctions in [0,1] with denominator up to $30$ by size, three consecutive ones are $\frac{13}{29},\frac9{20}, \frac{5}{11}.$ For the bin around $\frac{9}{20}$ I would take boundaries $\frac{13+9}{29+20}=\frac{22}{49}$ and $\frac{9+5}{20+11}=\frac{14}{31}.$ Note that the "center" $\frac9{20}$ is only about a bit more than a third of the way from the left endpoint to the right. This is because the $11$ pushes harder then the $29.$ This is one of the more extreme examples.
At any rate, the first graph below uses bins around the $277$ fractions in lowest terms $\frac{a}b$ with $1 \leq a \lt b \lt 30.$ Into the bins I put all $B=100806$ irrational fractional parts $\sqrt{t^2+k}-t$ for $1 \leq t \leq 2k$ and $1 \leq t \leq 316.$ The count $c$ is is then normalized to the ratio of $\frac{c}{B}$ to the expected value $\frac{B}{w}$ where $w$ is the width of the bin. I left a little space between bins. The normalized values are quite close to $1.$ The maximum discrepancy is $ 0.060188$ and the average is $0.01477$
The second graph is the same thing except each bin is split at the rational it surrounds. The left part is colored red and the right part green. It seems that usually, but not always, it is as with $\frac12,$ the left half is over $1$ and the rights half under. The variation is larger by a. The maximum deviations for the left and right halves are $.1763188$ and $.160645$ while the average discrepancies are $0.047085$ and $0.045767.$