The polynomial $P(x,y,z)=x^2+y^2+\frac{z^3}{3}+z$ has no critical values. Any level set $L_c := P^{-1}(c)$ is a surface of revolution around the $z$-axis. Here's an image of the $L_c$ for $c=-30,-15,0,15,30$ (drawn in SageMath):
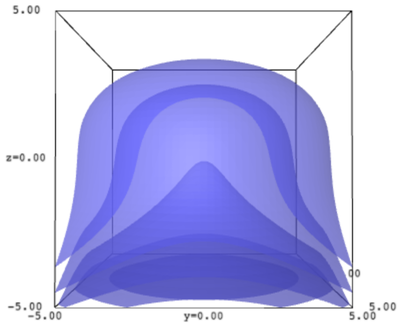 $L_c$ for $c=-30,-15,0,15,30$" />
$L_c$ for $c=-30,-15,0,15,30$" />
Actually, any level set $L_c$ has a unique Killing vector field $X_c$ which vanishvanishes just at one point $O_c$. Let $K(c)$ (resp. $LK(c)$) be the Gauss curvature of $L_c$ at $O_c$ (resp. the value of the Laplace-Beltrami operator applied to the Gauss curvature of $L_c$ at $O_c$). If $L_c$ and $L_{c'}$ are isometric then $K(c)=K(c')$ and $LK(c)=LK(c')$. Using a software I got that $K(c)=K(c')$ iff $c=-c'$. Again using the computer, I got $LK(c) \neq LK(-c)$ for all $c$. Here are the formulae, where $u=(\sqrt{9 c^2+4}+3 c)/2$: $$K(c) = \frac{4}{(u^{2/3}-1+u^{-2/3})^2}$$$$K(c) = \frac{4}{(u^{2/3}-1+u^{-2/3})^2},$$ $$LK(c)=\frac{128 \sqrt[3]{u} \left(-1-\sqrt[3]{u}+u^{2/3}\right) \left(2u+3 c+9c^2u\right)}{\sqrt{9 c^2+4} \left(1-u^{2/3}+u^{4/3}\right)^4}$$$$LK(c)=\frac{128 \sqrt[3]{u} \left(-1-\sqrt[3]{u}+u^{2/3}\right) \left(2u+3 c+9c^2u\right)}{\sqrt{9 c^2+4} \left(1-u^{2/3}+u^{4/3}\right)^4}.$$
