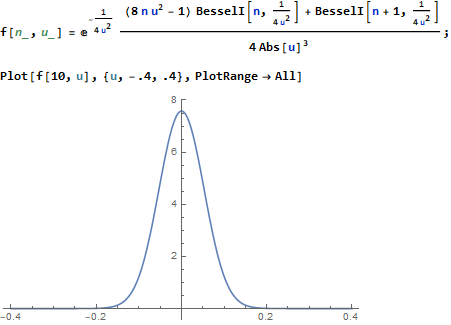
Addition in response to a comment by the OP: To find the pdf of $U=\Re z$, one can again use the referenced transformation technique. Indeed, without loss of generality, $U=\sqrt R\,C$, where $C:=\cos\Theta$ and $\Theta$ is a r.v. independent of $R$ and uniformly distributed in the interval $[0,2\pi]$ or, by symmetry, in $[0,\pi]$, so that for the pdf $f_C$ of $C$ one has $f_C(c)=\frac1\pi\,/\sqrt{1-c^2}$ for $-1<c<1$. Considering now the transformation from $(R,C)$ to $(U,W)$, where $U=\sqrt R\,C$ and $W=R$, we have the following rather complicated expression for the pdf of $U$: \begin{equation*} f_U(u)=\int_{u^2}^\infty \frac1{\pi\sqrt w}f_R(w)\frac{dw}{\sqrt{1-u^2/w}} =\frac1\pi\,\int_{u^2}^\infty \frac{f_R(w)\,dw}{\sqrt{w-u^2}} \end{equation*} for all real $u$, where $f_R$ is given by (1). Using the Fubini theorem and Mathematica for the calculation of the iterated double integral, we get \begin{equation*} f_U(u)=\exp\Big\{{-\frac{1}{4 u^2}}\Big\} \,\frac{\left(8 n u^2-1\right) I_n\left(\frac{1}{4 u^2}\right)+I_{n+1}\left(\frac{1}{4 u^2}\right)}{4 |u|^3}, \end{equation*} where $I_n(z)$ is the modified Bessel function of the first kind. HereBelow is the (bell-shaped) graph of $f_U=f_{\Re z}$ for $n=10$, which looks quite similar to your histogram. I am also including the corresponding Mathematica code: