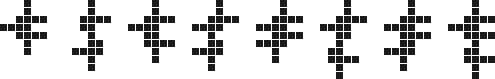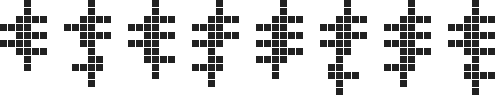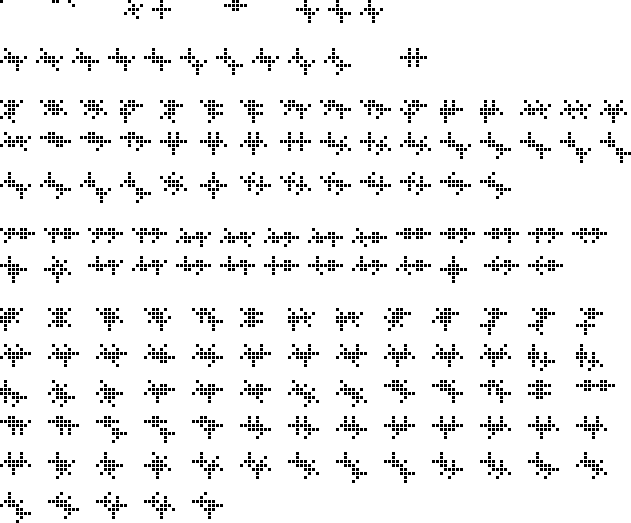Return to Answer

Ps. To answer a question posed in the comments, yes, vanishing $n$-plets (and, in fact, $n$-ominoes) exist for all $n \ge 14$. For example, the following family of vanishing 16 to 23 cell polyominoes:

$\hspace{82px}$

is easily extensible to all higher cell counts, as shown below for 24 to 32 cells:

$\hspace{82px}$

(In fact, even the 20 to 23 cell polyominoes above are just simple extensions of the 16 to 19 cell ones.) Together with the 14 and 15 cell polyominoes already found by the brute force search, these cover all the sizes from 14 cells up.

$$ \begin{array}{r|r} \text{cells} & \text{polyplets} \\ \hline 1 & 1 \\ 2 & 2 \\ 9 & 2 \\ 10 & 1 \\ 12 & 3 \\ 14 & 10 \end{array} \quad \begin{array}{r|r} \text{cells} & \text{polyplets} \\ \hline 15 & 1 \\ 16 & 45 \\ 17 & 27 \\ 18 & 70 \\ 19 & 98 \\ 20 & 285 \end{array} $$

$$ \begin{array}{r|r} \text{cells} & 1 & 2 & 9 & 10 & 12 & 14 & 15 & 16 & 17 & 18 & 19 & 20 \\ \hline \text{polyplets} & 1 & 2 & 2 & 1 & 3 & 10 & 1 & 45 & 27 & 70 & 98 & 285 \end{array} $$

Here's a picture by Sebastien showing all the 16-cell and smaller vanishing polyplets:

Here's a picture showing all the 18-cell and smaller vanishing polyplets:

$\hspace{14px}$