A $n$-polyplet is a collection of $n$ cells on a grid which are orthogonally or diagonally connected.
The number of $n$-polyplets is given by the OEIS sequence A030222: $1, 2, 5, 22, 94, 524, 3031, \dots$
See below the five $3$-polyplets:
$$
\substack{
\displaystyle{◻◻◻◻} \cr
\displaystyle{◻◼◻◻} \cr
\displaystyle{◻◼◼◻} \cr
\displaystyle{◻◻◻◻}
}
\quad \quad \quad
\substack{
\displaystyle{◻◻◻◻◻} \cr
\displaystyle{◻◼◼◼◻} \cr
\displaystyle{◻◻◻◻◻}
}
\quad \quad \quad
\substack{
\displaystyle{◻◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻} \cr
\displaystyle{◻◼◼◻◻} \cr
\displaystyle{◻◻◻◻◻}
}
\quad \quad \quad
\substack{
\displaystyle{◻◻◻◻◻} \cr
\displaystyle{◻◻◼◻◻} \cr
\displaystyle{◻◼◻◼◻} \cr
\displaystyle{◻◻◻◻◻}
}
\quad \quad \quad
\substack{
\displaystyle{◻◻◻◻◻} \cr
\displaystyle{◻◼◻◻◻} \cr
\displaystyle{◻◻◼◻◻} \cr
\displaystyle{◻◻◻◼◻} \cr
\displaystyle{◻◻◻◻◻}
}
$$
A
A polyplet will be called $1$-step vanishing on Conway's game of life, if every cell dies after one step.
We observed that for $n\le 4$, a $n$-polyplet is $1$-step vanishing iff $n \le 2$.
We found $1$-step vanishing polyplets with $n=9, 12$, see below:
$$
\substack{
\displaystyle{◻◻◻◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◻◻} \cr
\displaystyle{◻◻◻◼◻◻◻} \cr
\displaystyle{◻◼◼◼◼◼◻} \cr
\displaystyle{◻◻◻◼◻◻◻} \cr
\displaystyle{◻◻◻◼◻◻◻} \cr
\displaystyle{◻◻◻◻◻◻◻}
}
\quad \quad \quad \quad \quad \quad \quad \quad
\substack{
\displaystyle{◻◻◻◻◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◻◻◻} \cr
\displaystyle{◻◼◼◼◼◻◻◻} \cr
\displaystyle{◻◻◻◼◼◼◼◻} \cr
\displaystyle{◻◻◻◻◼◻◻◻} \cr
\displaystyle{◻◻◻◻◼◻◻◻} \cr
\displaystyle{◻◻◻◻◻◻◻◻}
}
$$

Question: Is there an other $1$-step vanishing $n$-polyplet with $n \le 12$?
If yes, what are they? (note that there are exactly $37963911$ $n$-polyplets with $n \le 12$).
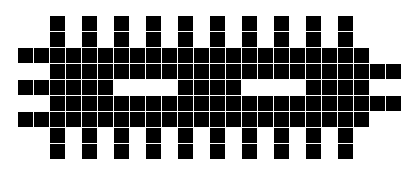
We can build infinitely many $1$-step vanishing polyplets with such pattern, see below with $n=142$:
$$
\substack{
\displaystyle{◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◻◻◻} \cr
\displaystyle{◻◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◻◻◻} \cr
\displaystyle{◻◻◻◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◻} \cr
\displaystyle{◻◼◼◼◼◼◼◻◻◻◻◼◼◼◼◻◻◻◻◼◼◼◼◻◻◻} \cr
\displaystyle{◻◻◻◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◻} \cr
\displaystyle{◻◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◼◻◻◻} \cr
\displaystyle{◻◻◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◻◻◻} \cr
\displaystyle{◻◻◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◼◻◻◻◻} \cr
\displaystyle{◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻◻}
}$$
Bonus question: Are there $1$-step vanishing polyplets of an other kind?



