Here are a few pictures and speculations to add to the the great ones from @j.c.
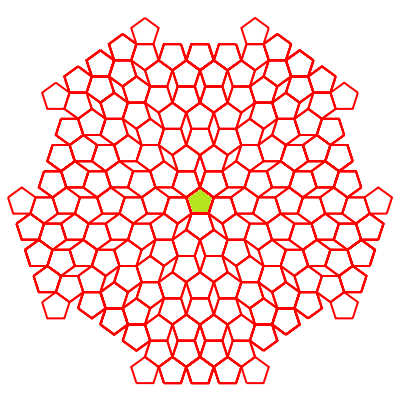
Here are the pentagons which arise from starting with the central green one and doing the reflections which do not overlap any previous ones.
These are just the outer frontiers of the $C_i.$ (Specifically, this shows the frontiers for $i \le 7$ and half the frontier for $i=8$i=8$.) It seems clear (though I do not claim a proof) that for a target point inside one of the pentagons one does best to roll out to it using just these pentagons. For a target point in one of the rhombi one should roll out to a neighboring pentagon and at the last step reflect over a previously unused (for reflections) edge. One can use one of the bordering pentagons closest to the center for the closer half of the rhombus and a small part of the further half.
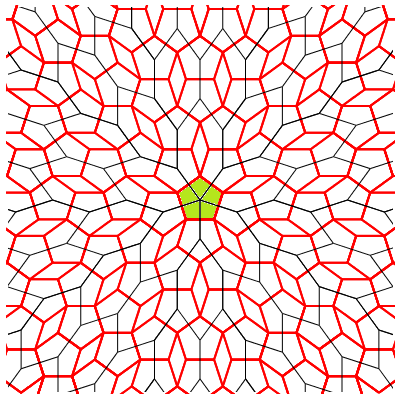
Here is a central portion of the same thing with lines connecting the centers of pentagons which share an edge. The resulting hexagons tile the plane with a $5$-fold rotational symmetry.
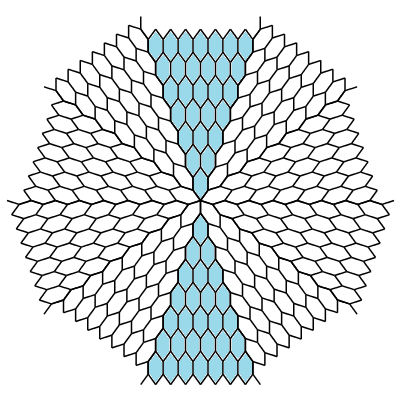
Finally, here is a picture of just these hexagons going out further. For each of the $5$ orientations, the hexagons of that orientation fall into $2$ connected components. One orientation is highlighted in blue.