Middle digits of the numbers $3^n$ are unpredictable. At least it is too hard for current techniques to say anything about them. It means that the their sum is unpredictable as well. Some good random number generators are based "digital" ideas.
If we take binary digits of $3^n$ then we immediately get generalization of $(3/2)^n$-problem which is out of reach today.
This picture is taken from a New Kind of Science: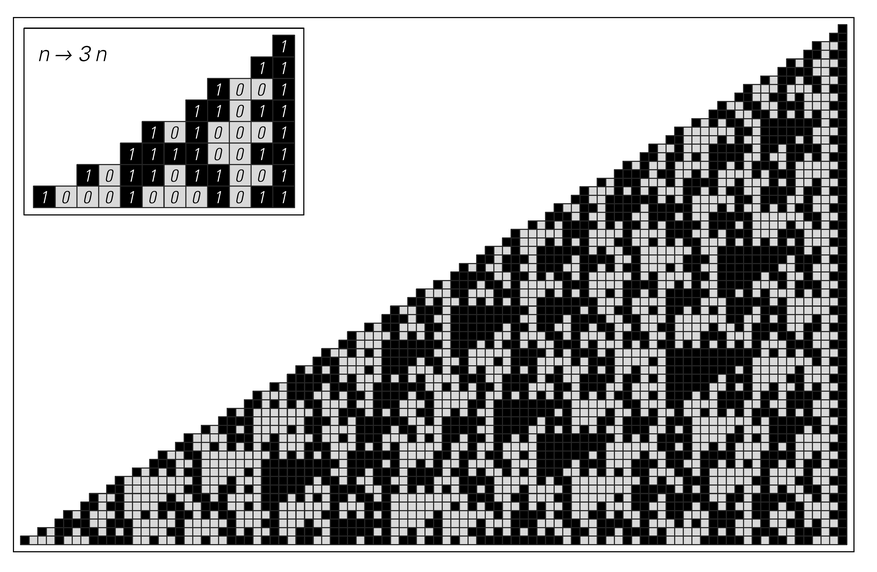
The pattern is very similar to "rule 30" picture from the same book: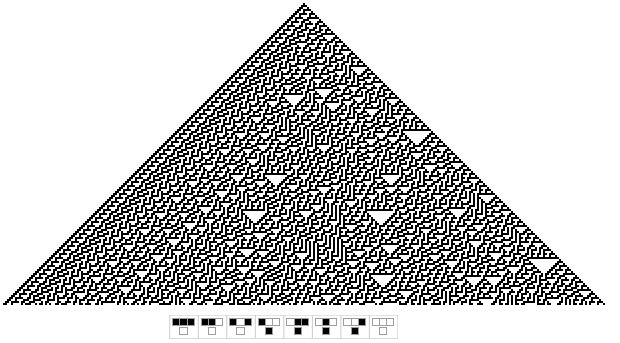
It is expected to have very good pseudorandom properties, see discussion at A New Kind of Science: A 15-Year View.
