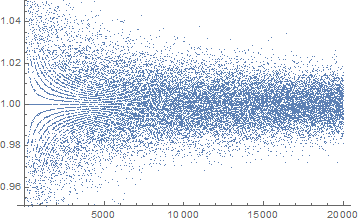
Experimentally, the sequence converges to $1,$ at the logarithmic rate suggested in Fedor's answer. Here is the graph for the first 20000 numbers:
Now, when we fit the actual $ds_{10}(3^m),$ we get the following suggestive graph:
Whose slope is pretty close to Fedor's "probabilistic"probabilistic" value. HOWEVER convergence is slow - empirically, Fedor's $o(1)$ term is actually of order $1/\log m.$